Not everyone knows that atmospheric pressure is different at different heights. There is even a special device for measuring both pressure and altitude. It's called a barometer- altimeter. In the article, we will study in detail how atmospheric pressure changes with height and what does air density have to do with it. Let's consider this dependence on the example of a graph.
Atmospheric pressure at different altitudes

Atmospheric pressure depends on altitude. When it is increased by 12 m, the pressure decreases by 1 mmHg. This fact can be written using the following mathematical expression: ∆h/∆P=12 m/mm Hg. Art. ∆h is the change in altitude, ∆P is the change in atmospheric pressure with a change in altitude by ∆h. What follows from this?
The formula shows how atmospheric pressure changes with altitude. So, if we rise by 12 m, then blood pressure will decrease by 12 mm Hg, if by 24 m - thenat 2 mmHg. Thus, by measuring atmospheric pressure, one can judge the height.
Millimeters of mercury and hectopascals
In some problems, pressure is expressed not in millimeters of mercury, but in pascals or hectopascals. Let us write the above relation for the case when the pressure is expressed in hectopascals. 1 mmHg Art.=133.3 Pa=1.333 hPa.
Now let's express the ratio of altitude and atmospheric pressure not in terms of millimeters of mercury, but in terms of hectopascals. ∆h/∆P=12 m/1, 333 hPa. After calculation we get: ∆h/∆P=9 m/hPa. It turns out that when we rise 9 meters, the pressure decreases by one hectopascal. Normal pressure is 1013 hPa. Let's round 1013 to 1000 and assume that this is exactly the BP on the surface of the Earth.
If we climb 90 meters, how does atmospheric pressure change with altitude? It decreases by 10 hPa, by 90 m - by 100 hPa, by 900 m - by 1000 hPa. If the pressure on the ground is 1000 hPa, and we climbed 900 m up, then the atmospheric pressure became zero. So, it turns out that the atmosphere ends at a nine-kilometer altitude? No. At such a height there is air, planes fly there. So what's the deal?
Relationship between air density and altitude. Features

How does atmospheric pressure change with height near the Earth's surface? The picture above has already answered this question. The higher the altitude, the lower the air density. As long as we are close to the surface of the earth, the change in air density is imperceptible. Therefore, for eachper unit height, the pressure decreases by about the same value. The two expressions we wrote down earlier should be taken as correct only if we are close to the surface of the Earth, not higher than 1-1.5 km.
A graph showing how atmospheric pressure changes with altitude
Now let's move on to visibility. Let's build a graph of atmospheric pressure versus altitude. At zero height P0=760mm Hg. Art. Due to the fact that with increasing altitude, the pressure decreases, atmospheric air will be less compressed, its density will become less. Therefore, on the graph, the dependence of pressure on height will not be described by a straight line. What does this mean?
How does atmospheric pressure change with altitude? Above the ground? At an altitude of 5.5 km, it decreases by 2 times (Р0/2). It turns out that if we rise to the same height, that is, 11 km, the pressure will decrease by another half and will be equal to Р0/4, etc.
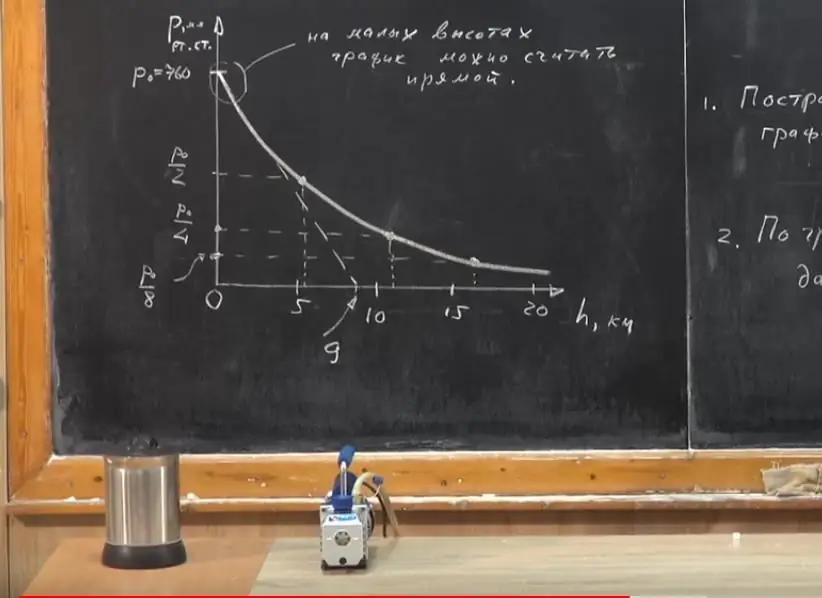
Let's connect the dots and we will see that the graph is not a straight line, but a curve. Why, when we wrote down the dependence relation, did it seem that the atmosphere ends at an altitude of 9 km? We considered that the graph is straight at any heights. This would be the case if the atmosphere were liquid, that is, if its density were constant.
It is important to understand that this graph is only a fragment of the dependence at low altitudes. At no point on this line does the pressure drop to zero. Even in deep space, there are gas molecules, which, however, do not haverelation to the earth's atmosphere. There is no absolute vacuum, emptiness in any point of the Universe.






