Judging by the popularity of the request "Fermat's theorem - a short proof", this mathematical problem is really of interest to many. This theorem was first stated by Pierre de Fermat in 1637 on the edge of a copy of the Arithmetic, where he claimed that he had a solution that was too large to fit on the edge.
The first successful proof was published in 1995 - it was the complete proof of Fermat's Theorem by Andrew Wiles. It has been described as "staggering progress" and led Wiles to receive the Abel Prize in 2016. Although described relatively briefly, the proof of Fermat's theorem also proved much of the modularity theorem and opened up new approaches to numerous other problems and effective methods for lifting modularity. These accomplishments have advanced mathematics 100 years into the future. The proof of Fermat's little theorem today is notis something out of the ordinary.

The unsolved problem stimulated the development of algebraic number theory in the 19th century and the search for a proof of the modularity theorem in the 20th century. This is one of the most notable theorems in the history of mathematics, and until the full division proof of Fermat's Last Theorem, it was in the Guinness Book of Records as "the most difficult mathematical problem", one of the features of which is that it has the largest number of unsuccessful proofs.
Historical background
Pythagorean equation x2 + y2=z2 has an infinite number of positive integer solutions for x, y and z. These solutions are known as Pythagorean trinities. Around 1637, Fermat wrote on the edge of the book that the more general equation a + b =chas no solutions in natural numbers if n is an integer greater than 2. Although Fermat himself claimed to have a solution to his problem, he did not leave any details about its proof. The elementary proof of Fermat's theorem, claimed by its creator, was rather his boastful invention. The book of the great French mathematician was discovered 30 years after his death. This equation, called Fermat's Last Theorem, remained unsolved in mathematics for three and a half centuries.
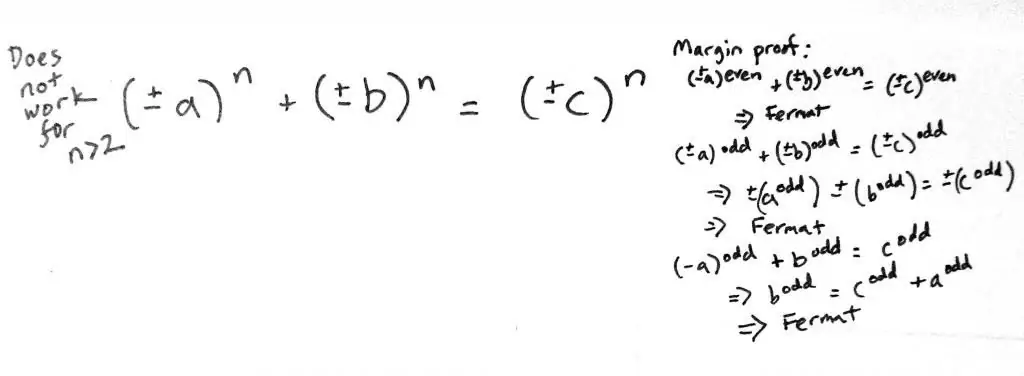
The theorem eventually became one of the most notable unsolved problems in mathematics. Attempts to prove this caused a significant development of number theory, and with the passagetime, Fermat's last theorem became known as an unsolved problem in mathematics.
A Brief History of Evidence
If n=4, as proved by Fermat himself, it suffices to prove the theorem for indices n that are prime numbers. Over the next two centuries (1637-1839) the conjecture was only proven for the primes 3, 5 and 7, although Sophie Germain updated and proved an approach that applied to the whole class of primes. In the mid-19th century, Ernst Kummer extended this and proved the theorem for all regular primes, whereby irregular primes were analyzed individually. Based on Kummer's work and using sophisticated computer research, other mathematicians were able to extend the solution of the theorem, with the goal of covering all the main exponents up to four million, but the proof for all exponents was still not available (meaning that mathematicians usually considered the solution of the theorem impossible, extremely difficult, or unattainable with current knowledge).
The work of Shimura and Taniyama
In 1955, Japanese mathematicians Goro Shimura and Yutaka Taniyama suspected that there was a connection between elliptic curves and modular forms, two very different branches of mathematics. Known at the time as the Taniyama-Shimura-Weyl conjecture and (ultimately) as the modularity theorem, it existed on its own, with no apparent connection to Fermat's last theorem. It itself was widely regarded as an important mathematical theorem, but it was considered (like Fermat's theorem) impossible to prove. At thatAt the same time, the proof of Fermat's Last Theorem (by dividing and applying complex mathematical formulas) was carried out only half a century later.
In 1984, Gerhard Frey noticed an obvious connection between these two previously unrelated and unsolved problems. A complete confirmation that the two theorems were closely related was published in 1986 by Ken Ribet, who based on a partial proof by Jean-Pierre Serra, who proved all but one part, known as the "epsilon hypothesis". Simply put, these works by Frey, Serra, and Ribe showed that if the modularity theorem could be proved, at least for a semistable class of elliptic curves, then the proof of Fermat's last theorem would sooner or later be discovered as well. Any solution that can contradict Fermat's last theorem can also be used to contradict the modularity theorem. Therefore, if the modularity theorem turned out to be true, then by definition there cannot be a solution that contradicts Fermat's last theorem, which means that it should have been proved soon.
Although both theorems were hard problems in mathematics, considered unsolvable, the work of the two Japanese was the first suggestion of how Fermat's last theorem could be extended and proven for all numbers, not just some. Important for the researchers who chose the topic of study was the fact that, in contrast to Fermat's last theorem, the modularity theorem was the main active area of research, for whichevidence was developed, and not just historical oddity, so the time spent on her work could be justified from a professional point of view. However, the general consensus was that the solution of the Taniyama-Shimura hypothesis was not feasible.
Farm's Last Theorem: Wiles' proof
Having learned that Ribet had proven Frey's theory correct, English mathematician Andrew Wiles, who has been interested in Fermat's Last Theorem since childhood and has experience working with elliptic curves and adjacent domains, decided to try to prove the Taniyama-Shimura Conjecture as a way to prove Fermat's Last Theorem. In 1993, six years after announcing his goal, while secretly working on the problem of solving the theorem, Wiles succeeded in proving a related conjecture, which in turn would help him prove Fermat's last theorem. Wiles' document was huge in size and scope.
A flaw was discovered in one part of his original paper during peer review and required another year of collaboration with Richard Taylor to jointly solve the theorem. As a result, Wiles' final proof of Fermat's Last Theorem was not long in coming. In 1995, it was published on a much smaller scale than Wiles's previous mathematical work, illustrating that he was not mistaken in his previous conclusions about the possibility of proving the theorem. Wiles' achievement was widely publicized in the popular press and popularized in books and television programs. The remaining parts of the Taniyama-Shimura-Weyl conjecture, which have now been proven andknown as the modularity theorem, were subsequently proven by other mathematicians who built on Wiles' work between 1996 and 2001. For his achievement, Wiles has been honored and received numerous awards, including the 2016 Abel Prize.
Wiles' proof of Fermat's last theorem is a special case of solving the modularity theorem for elliptic curves. However, this is the most famous case of such a large-scale mathematical operation. Along with solving Ribe's theorem, the British mathematician also obtained a proof of Fermat's last theorem. Fermat's Last Theorem and Modularity Theorem were almost universally considered unprovable by modern mathematicians, but Andrew Wiles was able to prove to the scientific world that even pundits can be wrong.
Wyles first announced his discovery on Wednesday 23 June 1993 at a Cambridge lecture titled "Modular Forms, Elliptic Curves and Galois Representations". However, in September 1993, it was found that his calculations contained an error. A year later, on September 19, 1994, in what he would call "the most important moment of his working life," Wiles stumbled upon a revelation that allowed him to fix the solution to the problem to the point where it could satisfy the mathematical community.
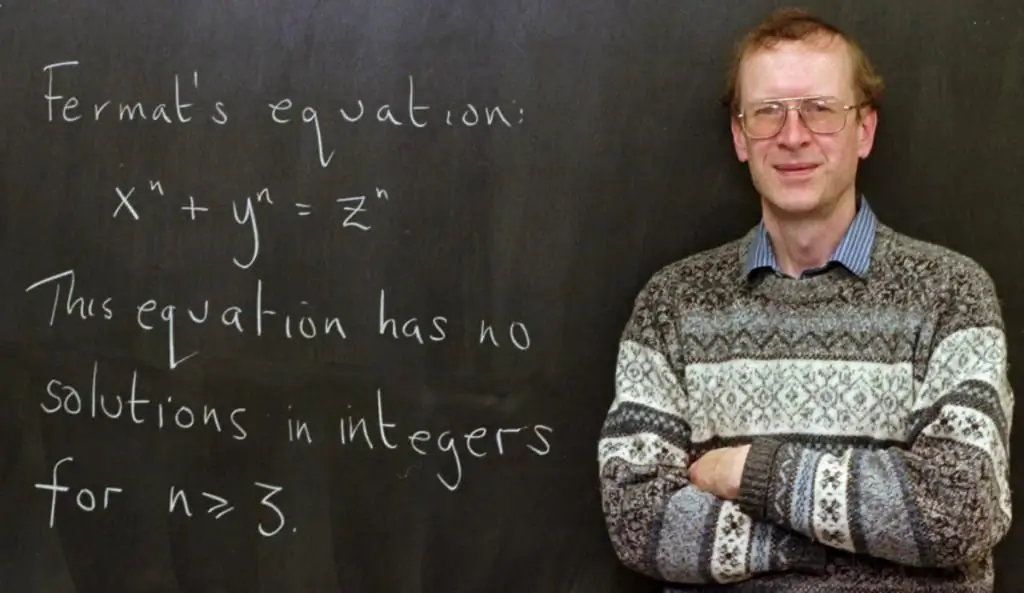
Work description
Proof of Fermat's Theorem by Andrew Wiles uses many methods from algebraic geometry and number theory and has many ramifications in theseareas of mathematics. He also uses the standard constructions of modern algebraic geometry, such as the category of schemes and the Iwasawa theory, as well as other methods of the 20th century that were not available to Pierre de Fermat.
The two articles containing the evidence are 129 pages long and were written over the course of seven years. John Coates described this discovery as one of the greatest achievements of number theory, and John Conway called it the major mathematical achievement of the 20th century. Wiles, in order to prove Fermat's last theorem by proving the modularity theorem for the special case of semistable elliptic curves, developed powerful methods for lifting modularity and opened up new approaches to numerous other problems. For solving Fermat's last theorem, he was knighted and received other awards. When it became known that Wiles had won the Abel Prize, the Norwegian Academy of Sciences described his achievement as "a delightful and elementary proof of Fermat's last theorem."
How it was
One of the people who reviewed Wiles' original manuscript with the solution to the theorem was Nick Katz. In the course of his review, he asked the Briton a number of clarifying questions that led Wiles to admit that his work clearly contains a gap. In one critical part of the proof, an error was made that gave an estimate for the order of a particular group: the Euler system used to extend the Kolyvagin and Flach method was incomplete. The mistake, however, did not make his work useless - every piece of Wiles's work was very significant and innovative in itself, as were manydevelopments and methods that he created in the course of his work and which affected only one part of the manuscript. However, this original work, published in 1993, did not really have a proof of Fermat's Last Theorem.

Wyles spent almost a year trying to rediscover the solution to the theorem, first alone and then in collaboration with his former student Richard Taylor, but all seemed to be in vain. By the end of 1993, rumors had circulated that Wiles's proof had failed in testing, but how serious that failure was was not known. Mathematicians began to put pressure on Wiles to reveal the details of his work, whether it was done or not, so that the wider community of mathematicians could explore and use whatever he was able to achieve. Instead of quickly correcting his mistake, Wiles only discovered additional difficult aspects in the proof of Fermat's Last Theorem, and finally realized how difficult it was.
Wyles states that on the morning of September 19, 1994, he was on the verge of giving up everything and giving up, and was almost resigned to failing. He was ready to publish his unfinished work so that others could build on it and find where he was wrong. The English mathematician decided to give himself one last chance and analyzed the theorem for the last time to try to understand the main reasons why his approach did not work, when he suddenly realized that the Kolyvagin-Flac approach would not work until hewill also include Iwasawa's theory in the proof process, making it work.
On October 6, Wiles asked three colleagues (including F altins) to review his new work, and on October 24, 1994, he submitted two manuscripts - "Modular elliptic curves and Fermat's last theorem" and "Theoretical properties of the ring of some Hecke algebras", the second of which Wiles co-wrote with Taylor and proved that certain conditions were met to justify the corrected step in the main article.
These two papers were reviewed and finally published as a full text edition in the May 1995 Annals of Mathematics. Andrew's new calculations were widely analyzed and eventually accepted by the scientific community. In these papers, the modularity theorem for semistable elliptic curves was established - the last step towards proving Fermat's Last Theorem, 358 years after it was created.
History of the Great Problem
Solving this theorem has been considered the biggest problem in mathematics for many centuries. In 1816 and in 1850 the French Academy of Sciences offered a prize for a general proof of Fermat's Last Theorem. In 1857, the Academy awarded 3,000 francs and a gold medal to Kummer for his research on ideal numbers, although he did not apply for the prize. Another prize was offered to him in 1883 by the Brussels Academy.
Wolfskell Prize
In 1908, the German industrialist and amateur mathematician Paul Wolfskel bequeathed 100,000 gold marks (a large amount for that time)Academy of Sciences of Göttingen, so that this money becomes a prize for the complete proof of Fermat's last theorem. On June 27, 1908, the Academy published nine award rules. Among other things, these rules required the proof to be published in a peer-reviewed journal. The prize was to be awarded only two years after publication. The contest was due to expire on September 13, 2007 - about a century after it began. On June 27, 1997, Wiles received Wolfschel's prize money and then another $50,000. In March 2016, he received €600,000 from the Norwegian government as part of the Abel Prize for "an amazing proof of Fermat's last theorem with the help of the modularity conjecture for semistable elliptic curves, opening a new era in number theory." It was the world triumph of the humble Englishman.

Before Wiles' proof, Fermat's theorem, as mentioned earlier, was considered absolutely unsolvable for centuries. Thousands of incorrect evidence at various times were presented to the Wolfskell committee, amounting to approximately 10 feet (3 meters) of correspondence. Only in the first year of the existence of the prize (1907-1908) 621 applications were submitted claiming to solve the theorem, although by the 1970s their number had decreased to about 3-4 applications per month. According to F. Schlichting, Wolfschel's reviewer, most of the evidence was based on elementary methods taught in schools and was often presented as "people with technical backgrounds but unsuccessful careers". According to the historian of mathematics Howard Aves, the lastFermat's theorem has set a kind of record - this is the theorem with the largest number of incorrect proofs.
Farm's laurels went to the Japanese
As mentioned earlier, around 1955, Japanese mathematicians Goro Shimura and Yutaka Taniyama discovered a possible connection between two apparently completely different branches of mathematics - elliptic curves and modular forms. The resulting modularity theorem (then known as the Taniyama-Shimura conjecture) states that every elliptic curve is modular, meaning that it can be associated with a unique modular form.
The theory was initially dismissed as unlikely or highly speculative, but was taken more seriously when number theorist André Weil found evidence to support the Japanese conclusions. As a result, the hypothesis has often been referred to as the Taniyama-Shimura-Weil hypothesis. She became part of the Langlands program, which is a list of important hypotheses that need to be proven in the future.
Even after serious scrutiny, the conjecture has been recognized by modern mathematicians as extremely difficult, or perhaps inaccessible to proof. Now this particular theorem is waiting for its Andrew Wiles, who could surprise the whole world with its solution.

Fermat's Theorem: Perelman's proof
Despite the popular myth, the Russian mathematician Grigory Perelman, for all his genius, has nothing to do with Fermat's theorem. Which, however, in no way detracts from it.numerous contributions to the scientific community.






