This article will explain the Black-Scholes formula in simple terms. The Black-Scholes model is a mathematical model of the dynamics of a financial market containing derivative investment instruments.
From the partial differential equation in the model (known as the Black-Scholes equation), the Black-Scholes formula can be derived. It gives a theoretical estimate of the price of European-style options and shows that the option has a unique price regardless of the security's risk and its expected return (instead of replacing the security's expected return with a risk-neutral rate).
The formula led to a boom in options trading and gave mathematical legitimacy to the Chicago Board Options Exchange and other options markets around the world. It is widely used, albeit often with adjustments and corrections, by options market participants. In the pictures in this article you can see examples of the Black-Scholes formula.

History and essence
Based on work previously developed by researchers and practitionersmarkets such as Louis Bachelier, Sheen Kassouf and Ed Thorpe, Fisher Black and Myron Scholes in the late 1960s demonstrated that dynamic portfolio revision eliminated the expected return of security.
In 1970, after they tried to apply the formula to the markets and suffered financial losses due to the lack of risk management in their professions, they decided to focus on their field, academia. After three years of effort, the formula, named after their promulgation, was finally published in 1973 in an article en titled "Pricing Options and Corporate Bonds" in the Journal of Political Economy. Robert S. Merton was the first to publish a paper expanding the mathematical understanding of the option pricing model and coined the term "Black-Scholes pricing model".
For their work, Merton and Scholes received the 1997 Nobel Memorial Prize in Economics, committee, citing their discovery of risk-independent dynamic revision as a breakthrough that decouples option from underlying security risk. Even though he did not receive the award due to his death in 1995, Black was mentioned by a Swedish academic as a participant. In the picture below you can see a typical Black-Scholes formula.
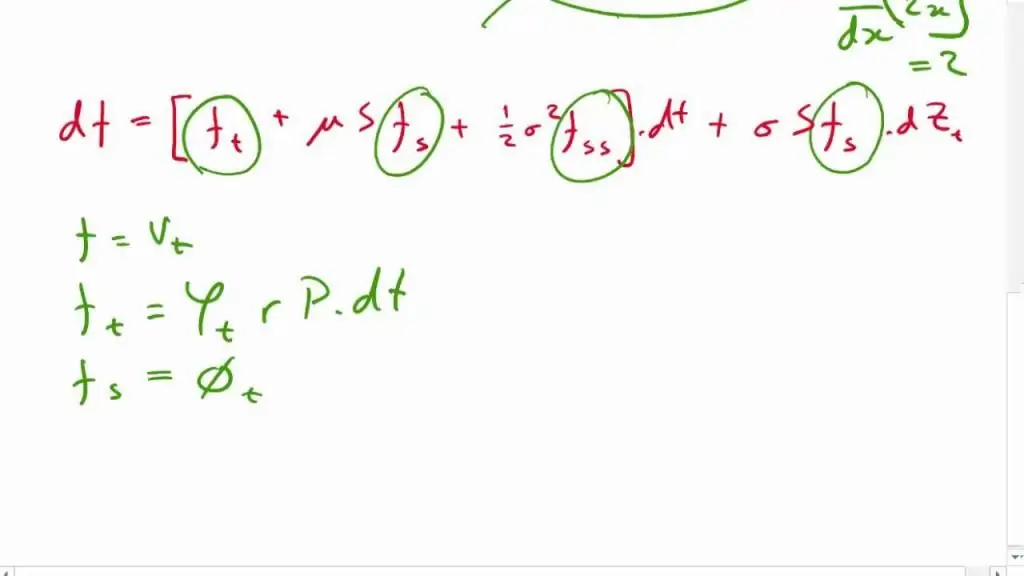
Options
The main idea of this model is to hedge an option by properly buying and selling the underlying asset and, as a result, eliminating the risk. This type of hedging is called "constantly updated delta hedging". Heis the basis for more complex strategies such as those used by investment banks and hedge funds.
Risk management
The assumptions of the model have been relaxed and generalized in many directions, resulting in a variety of models currently used in derivatives pricing and risk management. It is the understanding of the model, as shown in the Black-Scholes formula, that is often used by market participants, in contrast to actual prices. These details include no arbitrage limits and risk neutral pricing (due to constant review). In addition, the Black-Scholes equation, the partial differential equation that determines the price of an option, allows prices to be determined numerically when an explicit formula is not possible.

Volatility
The Black-Scholes formula has only one parameter that cannot be directly observed in the market: the average future volatility of the underlying asset, although it can be found at the price of other options. As the value of a parameter (whether put or call) increases in that parameter, it can be inverted to produce a "volatility surface" which is then used to calibrate other patterns such as OTC derivatives.
With these assumptions in mind, let's assume that derivatives are also traded in this market. We indicate that this security will have a certain payout on a certain date in the future, depending on the value assumed by the share.before this date. Surprisingly, the price of the derivative is now completely determined, although we do not know which path the share price will take in the future.
For a special case of a European call or put option, Black and Scholes showed that it was possible to create a hedged position consisting of a long position in a stock and a short position in an option, the value of which would not depend on the price of the stock. Their dynamic hedging strategy resulted in a partial differential equation that determined the price of the option. Its solution is given by the Black-Scholes formula.

Difference of terms
The Black-Scholes formula for excel can be interpreted by first splitting the call option into the difference of two binary options. A call option exchanges cash for an asset at expiration, while a call asset with or without an asset simply yields an asset (no cash in exchange) and a cashless call simply returns the money (no exchange of asset)). The Black-Scholes formula for an option is the difference of two terms, and these two terms are equal to the value of the binary call options. These binary options trade much less frequently than vanilla options, but are easier to analyze.
In practice, some sensitivity values are usually abbreviated to fit the scale of likely parameter changes. For example, rho divided by 10000 (change by 1 basis point), vega by 100 (change by 1 volume point) and theta by 365 are often reported.or 252 (1-day drawdown based on either calendar days or trading days per year).
The above model can be extended for variable (but deterministic) rates and volatility. The model can also be used to value European options for dividend payment instruments. In this case, closed-form solutions are available if the dividend is a known proportion of the share price. American and stock options that pay a known cash dividend (more realistic than a proportional dividend in the short term) are more difficult to value and a choice of solution methods (e.g. lattices and grids) is available.
Approach
Useful approximation: although volatility is not constant, model results often help set hedging in the right proportions to minimize risk. Even if the results are not entirely accurate, they serve as a first approximation to which adjustments can be made.

Basic for better models: The Black-Scholes model is robust in the sense that it can be adjusted to cope with some of its failures. Instead of treating some parameters (such as volatility or interest rates) as constants, we treat them as variables and thus add sources of risk.
This is reflected in the Greeks (changing the option value to change these parameters or equivalent to the partial derivatives with respect to these variables) and hedging these Greeksreduces the risk caused by the variable nature of these parameters. However, other defects cannot be eliminated by changing the model, in particular tail risk and liquidity risk, and instead they are managed outside the model, mainly by minimizing these risks and stress testing.
Explicit modeling
Explicit Modeling: This feature means that instead of assuming volatility a priori and calculating prices from it, you can use a model to determine volatility that gives the option's implied volatility at given prices, times and strike prices. By solving volatility over a given set of strike durations and prices, an implied volatility surface can be constructed.
In this application of the Black-Scholes model, a transformation of coordinates from the price area to the volatility area is obtained. Instead of quoting option prices in dollars per unit (which are difficult to compare based on strikes, durations, and coupon frequencies), option prices can be quoted in terms of implied volatility, leading to volatility trading in options markets.






