What is the phenomenon of superconductivity? Superconductivity is a phenomenon with zero electrical resistance and the release of magnetic flux fields that occur in certain materials, called superconductors, when cooled below a characteristic critical temperature.
The phenomenon was discovered by the Dutch physicist Heike Kamerling-Onnes on April 8, 1911 in Leiden. Like ferromagnetism and atomic spectral lines, superconductivity is a quantum mechanical phenomenon. It is characterized by the Meissner effect - a complete ejection of magnetic field lines from inside the superconductor during its transition to the superconducting state.
This is the essence of the phenomenon of superconductivity. The emergence of the Meissner effect indicates that superconductivity cannot be understood simply as an idealization of ideal conductivity in classical physics.
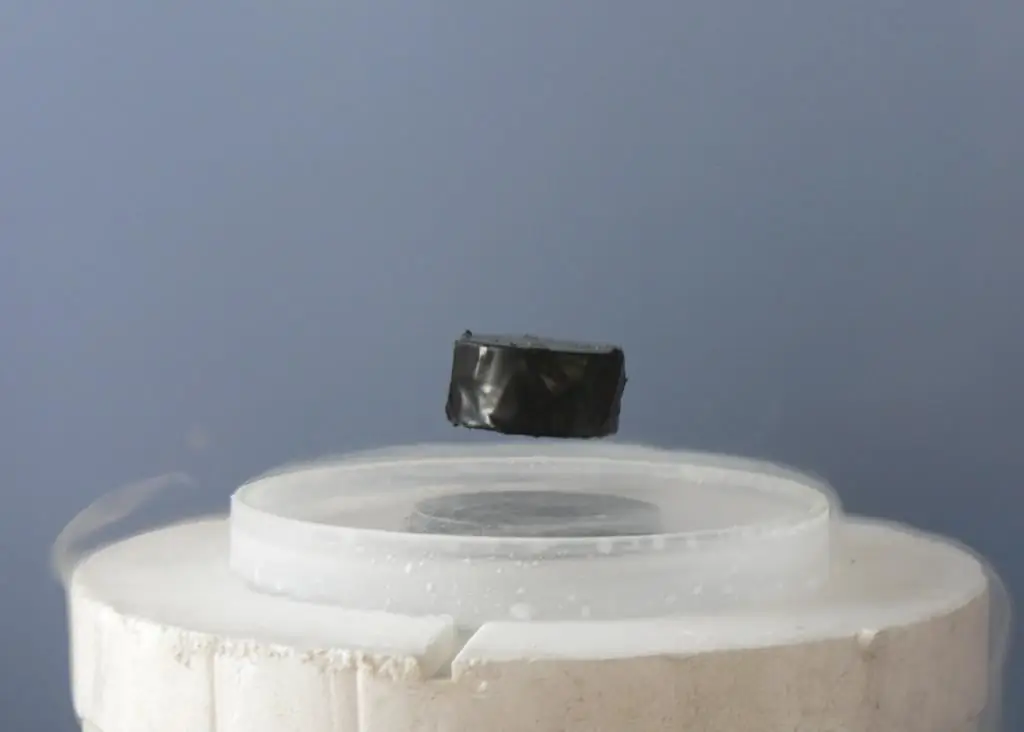
What is the phenomenon of superconductivity
The electrical resistance of a metal conductor gradually decreases aslowering the temperature. In common conductors such as copper or silver, this reduction is limited by impurities and other defects. Even near absolute zero, a real sample of a normal conductor shows some resistance. In a superconductor, the resistance drops sharply to zero when the material is cooled below its critical temperature. Electric current through a loop of superconducting wire can be maintained indefinitely without a power source. This is the answer to the question, what is the phenomenon of superconductivity.
History
In 1911, while studying the properties of matter at very low temperatures, Dutch physicist Heike Kamerling Onnes and his team discovered that the electrical resistance of mercury drops to zero below 4.2 K (-269°C). This was the very first observation of the phenomenon of superconductivity. Most chemical elements become superconducting at low enough temperatures.
Below a certain critical temperature, materials pass into a superconducting state, characterized by two main properties: first, they do not resist the passage of electric current. When resistance drops to zero, current can circulate within the material without energy dissipation.
Secondly, provided that they are sufficiently weak, external magnetic fields do not penetrate the superconductor, but remain on its surface. This field expulsion phenomenon became known as the Meissner effect after it was first observed by a physicist in 1933.
Three names, three letters and an incomplete theory
Ordinary physics does not give adequateexplanations of the superconducting state, as well as the elementary quantum theory of the solid state, which considers the behavior of electrons separately from the behavior of ions in a crystal lattice.
Only in 1957, three American researchers - John Bardeen, Leon Cooper and John Schrieffer created the microscopic theory of superconductivity. According to their BCS theory, electrons bunch into pairs through interaction with lattice vibrations (so-called "phonons"), thus forming Cooper pairs that move without friction inside a solid. A solid can be viewed as a lattice of positive ions immersed in a cloud of electrons. When an electron passes through this lattice, the ions move slightly, being attracted by the electron's negative charge. This movement generates an electrically positive region, which in turn attracts another electron.
The energy of the electronic interaction is quite weak, and vapors can be easily broken up by thermal energy - so superconductivity usually occurs at very low temperatures. However, the BCS theory does not provide an explanation for the existence of high-temperature superconductors at around 80 K (-193 °C) and above, for which other electron-binding mechanisms must be involved. The application of the phenomenon of superconductivity is based on the above process.
Temperature
In 1986, some cuprate-perovskite ceramic materials were found to have critical temperatures above 90 K (-183 °C). This high junction temperature is theoreticallyimpossible for a conventional superconductor, leading to materials being referred to as high-temperature superconductors. Available cooling liquid nitrogen boils at 77 K, and thus superconductivity at temperatures higher than these facilitates many experiments and applications that are less practical at lower temperatures. This is the answer to the question at what temperature does the phenomenon of superconductivity occur.
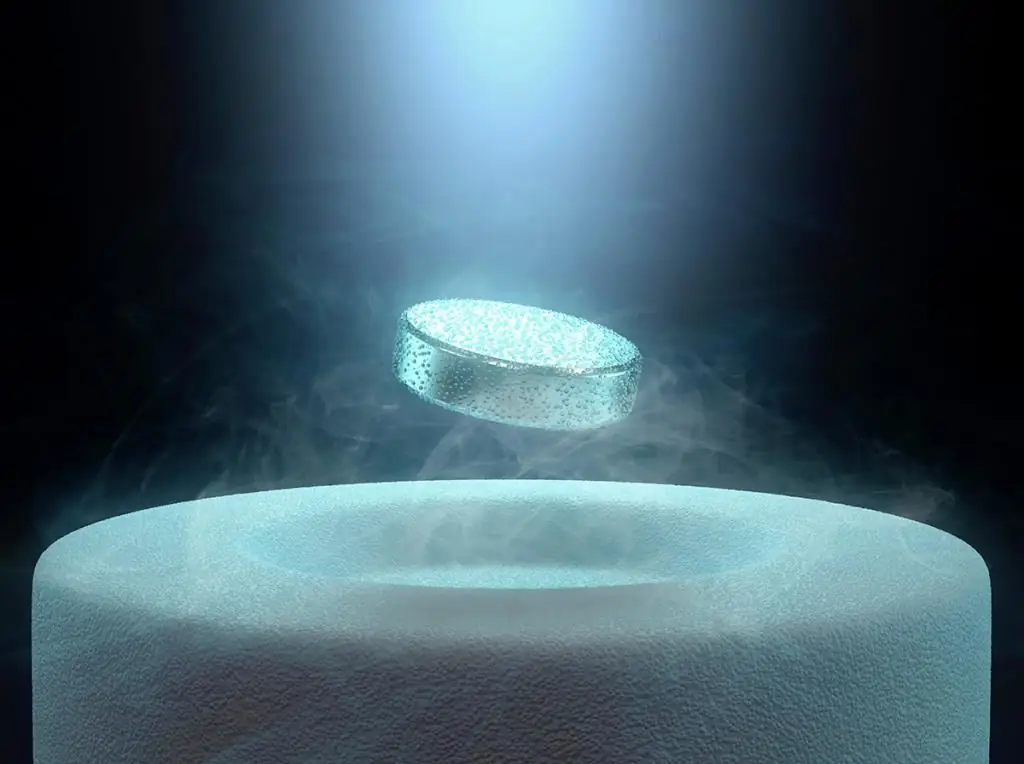
Classification
Superconductors can be classified according to several criteria that depend on our interest in their physical properties, on the understanding we have about them, on how expensive it is to cool them, or on the material they are made from.
By its magnetic properties
Type I superconductors: those that have only one critical field, Hc, and abruptly transition from one state to another when it is reached.
Type II superconductors: having two critical fields, Hc1 and Hc2, being perfect superconductors under the lower critical field (Hc1) and completely leaving the superconducting state above the upper critical field (Hc2), being in a mixed state between the critical fields.
As we understand them about them
Ordinary superconductors: those that can be fully explained by BCS theory or related theories.
Unconventional superconductors: those that could not be explained using such theories, for example: heavy fermionicsuperconductors.
This criterion is important because the BCS theory has been explaining the properties of conventional superconductors since 1957, but on the other hand, there has been no satisfactory theory to explain the completely unconventional superconductors. In most cases, Type I superconductors are common, but there are a few exceptions, such as niobium, which is both common and Type II.

By their critical temperature
Low temperature superconductors, or LTS: those whose critical temperature is below 30 K.
High temperature superconductors, or HTS: those whose critical temperature is above 30 K. Some now use 77 K as a separation to emphasize whether we can cool the sample with liquid nitrogen (whose boiling point is 77 K), which is much more feasible than liquid helium (an alternative to reach the temperatures needed to produce low temperature superconductors).
Other details
A superconductor can be type I, which means it has a single critical field, above which all superconductivity is lost, and below which the magnetic field is completely eliminated from the superconductor. Type II, meaning it has two critical fields between which it allows partial penetration of the magnetic field through isolated points. These points are called vortices. In addition, in multicomponent superconductors, a combination of two behaviors is possible. In this case, the superconductor is of type 1, 5.
Properties
Most of the physical properties of superconductors vary from material to material, such as heat capacity and critical temperature, critical field and critical current density at which superconductivity breaks down.
On the other hand, there is a class of properties that are independent of the base material. For example, all superconductors have absolutely zero resistivity at low applied currents, when there is no magnetic field, or when the applied field does not exceed a critical value.
The presence of these universal properties implies that superconductivity is a thermodynamic phase and therefore has certain distinctive properties that are largely independent of microscopic details.

The situation is different in the superconductor. In a conventional superconductor, the electron liquid cannot be separated into individual electrons. Instead, it consists of bound pairs of electrons known as Cooper pairs. This pairing is caused by the attractive force between electrons resulting from the exchange of phonons. Due to quantum mechanics, the energy spectrum of this liquid of the Cooper pair has an energy gap, that is, there is a minimum amount of energy ΔE that must be supplied to excite the liquid.
Therefore, if ΔE is greater than the thermal energy of the grating given by kT, where k is the Boltzmann constant and T is the temperature, the liquid will not be scattered by the grating. SoThus, the Cooper vapor liquid is superfluid, which means that it can flow without dissipating energy.

Superconductivity characteristics
In superconducting materials, superconductivity characteristics appear when the temperature T drops below the critical temperature Tc. The value of this critical temperature varies from material to material. Conventional superconductors typically have critical temperatures ranging from about 20 K to less than 1 K.
For example, solid mercury has a critical temperature of 4.2 K. As of 2015, the highest critical temperature found for a conventional superconductor is 203 K for H2S, although a high pressure of about 90 gigapascals was required. Cuprate superconductors can have much higher critical temperatures: YBa2Cu3O7, one of the first cuprate superconductors discovered, has a critical temperature of 92 K, and mercury-based cuprates with critical temperatures exceeding 130 K have been found. The explanation for these high critical temperatures remains unknown.
Electron pairing due to phonon exchanges explains superconductivity in conventional superconductors, but does not explain superconductivity in newer superconductors which have a very high critical temperature.
Magnetic fields
Similarly, at a fixed temperature below the critical temperature, superconducting materials stop superconducting when an external magnetic field is applied that is greater thancritical magnetic field. This is because the Gibbs free energy of the superconducting phase increases quadratically with the magnetic field, while the free energy of the normal phase is approximately independent of the magnetic field.
If the material is superconducting in the absence of a field, then the free energy of the superconducting phase is less than that of the normal phase, and therefore, for some finite value of the magnetic field (proportional to the square root of the difference in free energies at zero), the two free energies will be equal, and there will be a phase transition to the normal phase. More generally, a higher temperature and a stronger magnetic field result in a smaller proportion of superconducting electrons and therefore a greater depth of penetration into London of external magnetic fields and currents. The penetration depth becomes infinite at the phase transition.

Physical
The onset of superconductivity is accompanied by abrupt changes in various physical properties, which is the hallmark of a phase transition. For example, the electron heat capacity is proportional to the temperature in the normal (not superconducting) regime. At the superconducting transition, it experiences a jump and after that it ceases to be linear. At low temperatures, it changes instead of e−α/T for some constant α. This exponential behavior is one of the evidence for the existence of an energy gap.
Phase transition
The explanation of the phenomenon of superconductivity is quiteobviously. The order of the superconducting phase transition has been discussed for a long time. Experiments show that there is no second-order transition, that is, latent heat. However, in the presence of an external magnetic field, there is latent heat because the superconducting phase has a lower entropy, lower than the critical temperature, than the normal phase.
Experimentally demonstrated the following: when the magnetic field increases and goes beyond the critical field, the resulting phase transition leads to a decrease in the temperature of the superconducting material. The phenomenon of superconductivity has been briefly described above, now it's time to tell you something about the nuances of this important effect.

Calculations made in the 1970s showed that it could in fact be weaker than the first order due to the influence of long-range fluctuations in the electromagnetic field. In the 1980s, it was theoretically shown using disorder field theory, in which superconductor vortex lines play a major role, that the transition is second order in the type II mode and first order (i.e., latent heat) in the type I mode, and that the two regions are separated by a tricritical point.
The results were strongly confirmed by computer simulations in Monte Carlo. This played an important role in the study of the phenomenon of superconductivity. The work continues at the present time. The essence of the phenomenon of superconductivity is not fully understood and explained from the point of view of modern science.






