When a person was just learning to count, his fingers were enough to determine that two mammoths walking by the cave were smaller than that herd behind the mountain. But as soon as he realized what positional reckoning is (when a number has a specific place in a long series), he began to think: what's next, what is the largest number?

Since then, the best minds have been looking for how to calculate such quantities, and most importantly, what meaning to give them.
Ellipsis at the end of the row
When schoolchildren are introduced to the initial concept of natural numbers, it is prudent to put dots along the edges of a series of numbers and explain that the largest and smallest numbers are a meaningless category. It is always possible to add one to the largest number, and it will no longer be the largest. But progress would not have been possible if there were not those willing to find meaning where there shouldn't be.
The infinity of the number series, in addition to a frightening and indefinite philosophical meaning, also created purely technical difficulties. I had to look for notation for very large numbers. At first, this was done separately for the mainlanguage groups, and with the development of globalization, words that name the largest number have appeared that are generally accepted around the world.
Ten, hundred, thousand
Every language has its own name for numbers of practical importance.
In Russian, first of all, it is a series from zero to ten. Up to a hundred, further numbers are called either on their basis, with a slight change in the roots - “twenty” (two by ten), “thirty” (three by ten), etc., or are composite: “twenty-one”, “fifty-four ". Exception - instead of "four" we have a more convenient "forty".
The largest two-digit number - "ninety-nine" - has a compound name. Further from their own traditional names - "one hundred" and "thousand", the rest are formed from the necessary combinations. The situation is similar in other common languages. It is logical to think that established names were given to numbers and figures that most ordinary people de alt with. Even an ordinary peasant could imagine what a thousand head of cattle is. With a million, it was more difficult, and confusion began.
Million, quintillion, decibillion
In the middle of the 15th century, the Frenchman Nicolas Chouquet, in order to designate the largest number, proposed a naming system based on numerals from the generally accepted Latin among scientists. In Russian, they have undergone some modification for ease of pronunciation:
- 1 - Unus - un.
- 2 - Duo, Bi (double) - duo, bi.
- 3 - Tres - three.
- 4 - Quattuor - quadri.
- 5 - Quinque - quinty.
- 6 - Sex - sexty.
- 7 - Septem -septi.
- 8 - Octo - Oct.
- 9 - Novem - noni.
- 10 - Decem - deci.
The basis of the names was supposed to be -million, from "million" - "big thousand" - i.e. 1 000 000 - 1000^2 - one thousand squared. This word, to mention the largest number, was first used by the famous navigator and scientist Marco Polo. So, a thousand to the third power became a trillion, 1000 ^ 4 became a quadrillion. Another Frenchman - Peletier - proposed for the numbers that Schuke called "thousand millions" (10^9), "thousand billions" (10^15) , etc., to use the ending "-billion". It turned out that 1,000,000,000 is a billion, 10^15is a billiard, a unit with 21 zeros is a trillion, and so on.
The terminology of French mathematicians began to be used in many countries. But it gradually became clear that 10^9in some works began to be called not a billion, but a billion. And in the USA they adopted a system according to which the ending -million received degrees not of a million, like the French, but of thousands. As a result, there are two scales in the world today: “long” and “short”. To understand what number is meant by the name, for example, a quadrillion, it is better to clarify to what degree the number 10 is raised. including in Russia (however, we have 10^9 - not a billion, but a billion), if in 24 - this is the "long", adopted in most regions of the world.
Tredecillion, vigintilliard and millillion
After the last numeral is used - deci, and it formsdecillion - the largest number without complex word formations - 10 ^ 33 on a short scale, combinations of the necessary prefixes are used for the following digits. It turns out complex compound names such as tredecillion - 10 ^ 42, quindecillion - 10 ^ 48, etc. The Romans were awarded non-compound, their own names: twenty - viginti, one hundred - centum and one thousand - mille. Following the rules of Shuquet, one can form monster names for an infinitely long time. For example, the number 10 ^308760 is called decentduomylianongentnovemdecillion.
But these constructions are of interest only to a limited number of people - they are not used in practice, and these quantities themselves are not even tied to theoretical problems or theorems. It is for purely theoretical constructions that giant numbers are intended, sometimes given very sonorous names or called by the author's last name.
Darkness, legion, asankheyya
The question of huge numbers also worried the “pre-computer” generations. The Slavs had several number systems, in some they reached great heights: the largest number is 10 ^ 50. From the heights of our time, the names of numbers seem like poetry, and only historians and linguists know whether all of them had a practical meaning: 10 ^ 4 - "darkness", 10 ^ 5 - "legion", 10 ^ 6 - "leodr", 10 ^7 - crow, raven, 10^8 - "deck".
An equally beautiful number asaṃkhyeya is mentioned in Buddhist texts, in ancient Chinese and ancient Indian collections of sutras.

The researchers give the quantitative value of the Asankheyya number as 10^140. For those who understand it is completedivine meaning: that is how many cosmic cycles the soul must go through in order to cleanse itself of everything bodily, accumulated over a long path of rebirth, and achieve the blissful state of nirvana.
Google, googolplex
A mathematician from Columbia University (USA) Edward Kasner from the early 1920s began to think about large numbers. In particular, he was interested in a sonorous and expressive name for the beautiful number 10^100. One day he was walking with his nephews and told them about this number. Nine-year-old Milton Sirotta suggested the word googol - googol. The uncle also received a bonus from his nephews - a new number, which they explained as follows: one and as many zeros as you can write until you get completely tired. The name of this number was googolplex. On reflection, Kashner decided that it would be the number 10^googol.
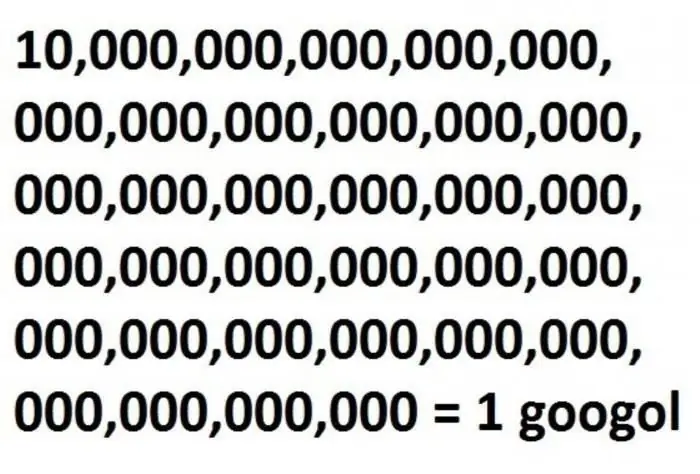
Kashner saw the meaning in such numbers more pedagogically: science did not know anything in such quantity at that time, and he explained to future mathematicians, using their example, what is the largest number that can keep the difference from infinity.
The smart idea of the little geniuses of naming was appreciated by the founders of the company promoting the new search engine. The googol domain was taken, and the letter o dropped out, but a name appeared for which an ephemeral number could someday become real - that's how much its shares will cost.
Shannon's number, Skuse's number, mezzon, megiston
Unlike physicists, who periodically stumble upon the limitations imposed by nature, mathematicians continue on their way towards infinity. Chess enthusiastClaude Shannon (1916-2001) gave meaning to the number 10^118 - this is how many variants of positions can appear within 40 moves.
Stanley Skewes from South Africa was working on one of the seven problems included in the list of "millennium problems" - the Riemann hypothesis. It concerns the search for patterns in the distribution of prime numbers. In the course of reasoning, he first used the number 10^10^10^34, designated by him as Sk1 , and then 10^10^10^963 - Skuse's second number - Sk 2.

Even the usual writing system is not suitable for operating with such numbers. Hugo Steinhaus (1887-1972) suggested using geometric shapes: n in a triangle is n to the power of n, n squared is n in n triangles, n in a circle is n in n squares. He explained this system using the example of numbers mega - 2 in a circle, mezzon - 3 in a circle, megiston - 10 in a circle. It is so difficult to designate, for example, the largest two-digit number, but it has become easier to operate with colossal values.
Professor Donald Knuth proposed arrow notation, in which repeated exponentiation was denoted by an arrow, borrowed from the practice of programmers. The googol in this case looks like 10↑10↑2, and the googolplex looks like 10↑10↑10↑2.
Graham's number
Ronald Graham (b. 1935), an American mathematician, in the course of studying the Ramsey theory associated with hypercubes - multidimensional geometric bodies - introduced special numbers G1 - G64, with which he marked the boundaries of the solution, where the upper limit was the largest multiple,named after him. He even calculated the last 20 digits, and the following values served as the initial data:
- G1=3↑↑↑↑3=8, 7 x 10^115.
- G2=3↑…↑3 (number of superpower arrows=G1).
- G3=3↑…↑3 (number of superpower arrows=G2).
- G64=3↑…↑3 (number of superpower arrows=G63)
G64, simply referred to as G, is the world's largest number used in mathematical calculations. It is listed in the book of records.

It is almost impossible to imagine its scale, given that the entire volume of the universe known to man, expressed in the smallest unit of volume (a cube with a Planck length face (10-35 m)), expressed as 10^185.






