The concept of a degree in mathematics is introduced in the 7th grade at the algebra lesson. And in the future, throughout the course of studying mathematics, this concept is actively used in its various forms. Degrees are a rather difficult topic, requiring memorization of values and the ability to correctly and quickly count. For faster and better work with mathematics degrees, they came up with the properties of a degree. They help to cut down on big calculations, to convert a huge example into a single number to some extent. There are not so many properties, and all of them are easy to remember and apply in practice. Therefore, the article discusses the main properties of the degree, as well as where they apply.

Degree properties
We will consider 12 properties of degrees, including properties of degrees with the same bases, and give an example for each property. Each of these properties will help you solve problems with degrees faster, as well as save you from numerous computational errors.
1st property.
a0=1
Many often forget about this property, doerrors by representing a number to the power of zero as zero.
2nd property.
a1=a
3rd property.
a am=a(n+m)
You need to remember that this property can only be used when multiplying numbers, it does not work with the sum! And don't forget that this and the following properties only apply to powers with the same base.
4th property.
a/am=a(n-m)
If the number in the denominator is raised to a negative power, then when subtracting, the degree of the denominator is taken in brackets to correctly replace the sign in further calculations.
Property works only for division, not for subtraction!
5th property.
(a)m=a(nm)
6th property.
a-n=1/a
This property can also be applied in reverse. A unit divided by a number to some degree is that number to a negative power.
7th property.
(ab)m=am bm
This property cannot be applied to sum and difference! When raising a sum or difference to a power, abbreviated multiplication formulas are used, not the properties of the power.
8th property.
(a/b)=a/b
9th property.
a½=√a
This property works for any fractional power with a numerator equal to one,the formula will be the same, only the degree of the root will change depending on the denominator of the degree.
Also, this property is often used in reverse. The root of any power of a number can be represented as that number to the power of one divided by the power of the root. This property is very useful in cases where the root of the number is not extracted.
10th property.
(√a)2=a
This property does not only work with square roots and second powers. If the degree of the root and the degree to which this root is raised are the same, then the answer will be a radical expression.
11th property.
√a=a
You need to be able to see this property in time when solving in order to save yourself from huge calculations.
12th property.
am/n=√am
Each of these properties will meet you more than once in tasks, it can be given in its pure form, or it may require some transformations and the use of other formulas. Therefore, for the correct solution, it is not enough to know only the properties, you need to practice and connect the rest of mathematical knowledge.
Using degrees and their properties
They are actively used in algebra and geometry. Degrees in mathematics have a separate, important place. With their help, exponential equations and inequalities are solved, as well as powers often complicate equations and examples related to other sections of mathematics. Exponents help to avoid large and long calculations, it is easier to reduce and calculate the exponents. But forworking with large powers, or with powers of large numbers, you need to know not only the properties of the degree, but also competently work with the bases, be able to decompose them in order to make your task easier. For convenience, you should also know the meaning of numbers raised to a power. This will reduce your time in solving by eliminating the need for long calculations.
The concept of degree plays a special role in logarithms. Since the logarithm, in essence, is the power of a number.
Reduced multiplication formulas are another example of using powers. They cannot use the properties of degrees, they are decomposed according to special rules, but in each abbreviated multiplication formula there are invariably degrees.
Degrees are also actively used in physics and computer science. All translations into the SI system are made using degrees, and in the future, when solving problems, the properties of the degree are applied. In computer science, powers of two are actively used, for the convenience of counting and simplifying the perception of numbers. Further calculations on the conversion of units of measurement or calculations of problems, just like in physics, occur using the properties of the degree.
Degrees are also very useful in astronomy, where you rarely see the use of the properties of a degree, but the degrees themselves are actively used to shorten the recording of various quantities and distances.
Degrees are also used in everyday life, when calculating areas, volumes, distances.
With the help of degrees, very large and very small quantities are written in any field of science.
Exponential equations and inequalities
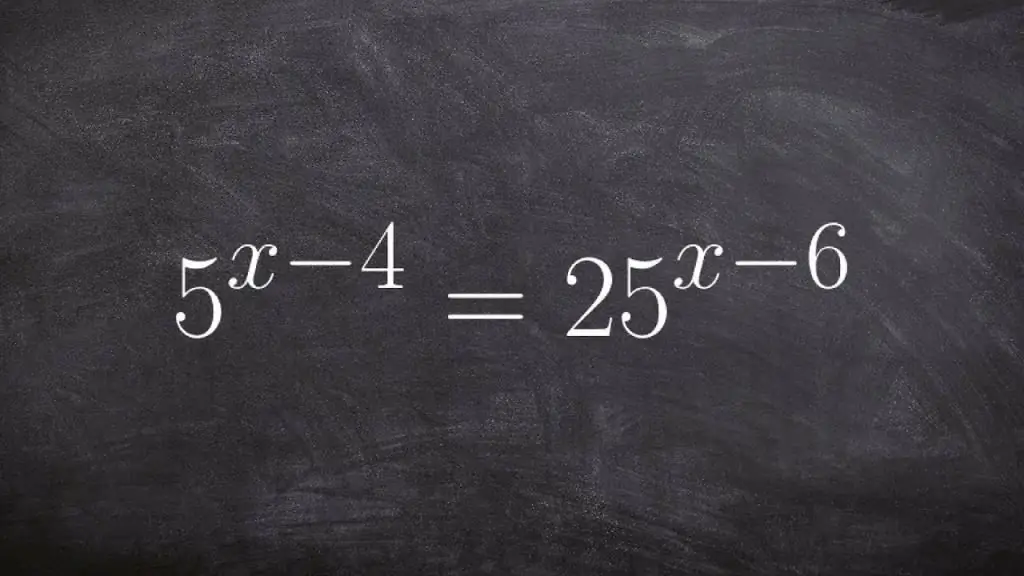
The degree properties occupy a special place precisely in exponential equations and inequalities. These tasks are very common, both in the school course and in exams. All of them are solved by applying the properties of the degree. The unknown is always in the degree itself, therefore, knowing all the properties, it will not be difficult to solve such an equation or inequality.






