When studying physics in grade 10, the topic of dipoles is considered. What does this concept mean and what formulas are used to calculate it?
Introduction
If you place a dipole in the space of a uniform electric field, you can represent it as lines of force. A dipole is a system in which there are two charges that are identical in parameters, but they are opposite point charges. Moreover, the distance between them will be much less than the distance to any point of the dipole field. The concept of the dipole moment is studied in the school course of electrodynamics (Grade 10).
The axis of the dipole is a straight line that passes through the points of both charges. A dipole arm is a vector that connects a charge and at the same time goes from negatively charged particles to positively charged particles. An electric dipole is characterized by the presence of such a state as a dipole or electric moment.
By definition, a dipole moment is a vector that is numerically equal to the product of the dipole charge and its arm. Moreover, it is co-directed with the shoulder of the dipole. At zero equality of the sum of forces, we calculate the value of the moment. For the angle that exists between the dipole moment anddirectionality of the electric field, the presence of a mechanical moment is characteristic.
Often people find it difficult to calculate the modulus acting on the dipole structure. Here it is necessary to take into account the peculiarities of calculating the angle "Alpha". It is known that the dipole deviates from the balanced position. But the dipole moment itself has a restoring character, as it tends to be in motion.
Calculations
When this dipole moment is placed in the medium of an inhomogeneous electric field, a force inevitably arises. In such an environment, the indicators of the sum of forces will not be zero. Consequently, there are forces acting on the dipole moment with a point character. The size of the dipole arm is much smaller.
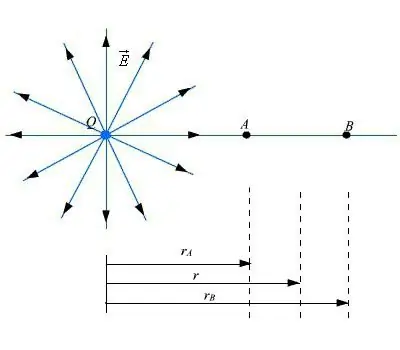
The formula can be written like this: F=q (E2 - E1)=qdE, where d is the electric field differential.
Search for the characteristics of the physical concept under study
Let's look at the topic further. In order to determine what is the characteristic of the electric field, if it is created using a system of charges and localized in a small space, it is necessary to make a number of calculations. An example is presented by atoms and molecules, which in their composition have electrically charged nuclei and electrons.
If it is necessary to search for a field at a distance greater than the dimensions that make up the area where the particles are located, we will use a number of exact formulas that are highly complex. It is possible to use simplerapproximate expressions. Let us assume that point sets of charges qk take part in the creation of the electric field. They are located in a small space.
To perform the calculation of the characteristic that the field has, it is allowed to combine all the charges of the system. Such a system is considered as a point charge Q. The magnitude indicators will be the sum of the charges that were in the original system.
Location of charges
Let's imagine that the location of the charge is indicated in any place where the system of charges qk is located. When making changes to the location, if it has limits expressed in a small area, such an influence will be negligible, almost imperceptible to the field at the point of view. Within such limits of approximation of strength and potential that the electric field has, determinations are made using traditional formulas.
When the sum of the total charge of the system is zero, the parameters of the indicated approximation will look rough. This gives reason to conclude that the electric field is simply absent. If it is necessary to obtain a more accurate approximation, mentally collect separate groups of positive and negative charges of the system that is being considered.
In the case of displacement of their "centers" relative to others, the field parameters in such a system can be described as a field that has two point charges, equal in magnitude and opposite in sign. It is noted that they are displaced in relation to others. To provideFor a more accurate characterization of the system of charges in terms of the parameters of this approximation, it will be necessary to study the properties of a dipole in an electric field.
Introduction of the term
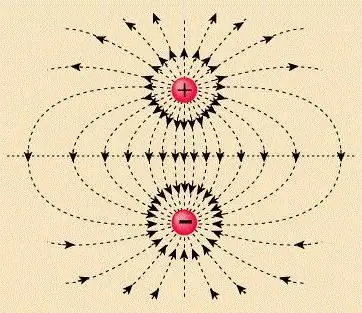
Let's get back to the definition. An electric dipole is the definition of a system that has two point charges. They have the same size and opposite signs. Moreover, such signs are located at small distances relative to other signs.
You can calculate the characteristic of the process that is created by means of a dipole, and it is represented by two point charges: +q and −q, and they are located at a distance a relative to the others.
Sequence of calculations
Let's start by calculating the potential and intensity that the dipole has on its axial surface. This is a straight line that runs between two charges. Provided that point A is located at a distance that is equal to r relative to the central part of the dipole, and if it is r >> a, according to the principle of superposition for the field potential at this point, it will be rational to use the expression to calculate the parameters of the electric dipole.
The magnitude of the strength vector is calculated by the principle of superposition. To calculate the field strength, the concept of the ratio of potential and field strength is used:
Ex=−Δφ /Δx.
Under such conditions, the direction of the intensity vector is indicated longitudinally relative to the dipole axis. To calculate its modulus, the standard formula is applicable.
Importantclarifications
It should be taken into account that the weakening of the electric dipole field occurs faster than it experiences a point charge. The decay of the dipole field potential is inversely proportional to the square of the distance, and the field strength is inversely proportional to the cube of the distance.
Using similar, but more cumbersome methods, the parameters of the potential and field strength of the dipole are found at arbitrary points, the position parameters of which are determined using such a calculation method as polar coordinates: the distance to the center of the electric dipole (r) and the angle (θ).
Calculation using tension vector
The concept of the intensity vector E is divided into two points:
- Radial (Er), which is directed in the longitudinal direction relative to the straight line.
- Such a straight line connects the specified point and the center of the dipole with the perpendicular to it Eθ.
This decomposition of each component is directed along the course of change that occurs with all coordinates of the point to be observed. You can find it by the ratio that relates field strength indicators to potential modifications.
Finding the vector component at the field strength, it is important to establish the nature of the relationship in the potential changes that occur due to the displacement of the observation point in the direction of the vectors.
Calculate the perpendicular component
When doneFor this procedure, it is important to take into account that the expression for the magnitude at a small perpendicular displacement will be determined by changing the angle: Δl=rΔθ. The magnitude parameters for this field component will be equal.
Having obtained the ratio, it is possible to determine the field of the electric dipole at an arbitrary point to build a picture with the lines of force of this field.
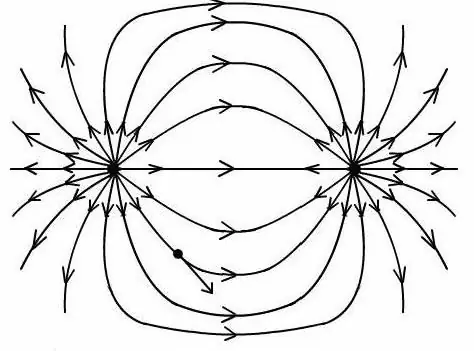
It is important to consider that all formulas for determining the potential and field strength of a dipole operate only on the product of the values \u200b\u200bthat one dipole charge has and the distance between them.
Dipole moment
The title of the described work is a complete description of the electrical type of properties. It has the name "dipole moment of the system".
By definition of a dipole, which is a system of point charges, it can be found out that it is characterized by the presence of axial symmetry, when the axis is a straight line that passes through several charges.
To set the full characteristic of the dipole, indicate the orientation direction that the axis has. For simplicity of calculations, the dipole moment vector can be specified. The value of its magnitude is equal to the magnitude of the dipole moment, and the direction vector differs by the coincidence of it and the axis of the dipole. So, p=qa if a is the direction of the vector that connects the negative and positive charges of the dipole.
The use of such a characteristic of the dipole is convenient and makes it possible in most cases to simplify the formula and give it the formvector. The description of the potential of the dipole field at a point of an arbitrary direction is written in the form of a vector.
The introduction of such concepts as the vector characteristic of a dipole and its dipole moment can be performed using a simplified model − a point charge in a uniform field, which includes a system of charges, the geometric dimensions of which do not have to be taken into account, but it is important to know the dipole moment. This is a prerequisite for performing calculations.
How a dipole behaves
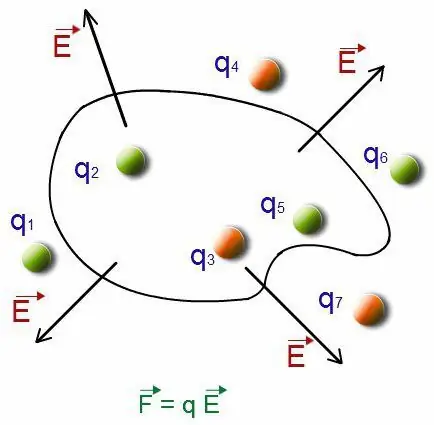
The behavior of a dipole can be seen on the example of such a situation. The position of two point charges has a fixed character of distance relative to each other. They were placed in the conditions of a dipole of a uniform electric field. Made observations on the process. In the lessons of physics (electrodynamics), this concept is considered in detail. From the field to the charge, the action of forces is performed:
F=±qE
They are equal in magnitude and opposite in direction. The indicator of the total force that acts on the dipole is zero. Since such a force has an effect on various points, the total moment will be:
M=Fa sin a=qEa sin a=pE sin a
with α being the angle connecting the field strength vectors and the dipole moment vectors. Due to the presence of a force moment, the dipole moment of the system tends to return to the directions of the electric field strength vector.
Electric dipole is a concept that is important to understand clearly. You can read more about it on the Internet. Also it canto study in physics lessons at school in grade 10, as we talked about above.






