Many people may be surprised by the fact that air has a certain non-zero weight. The exact value of this weight is not so easy to determine, since it is strongly influenced by factors such as chemical composition, humidity, temperature and pressure. Let's take a closer look at the question of how much air weighs.
What is air

Before answering the question of how much air weighs, it is necessary to understand what this substance is. Air is a gas envelope that exists around our planet, and which is a homogeneous mixture of various gases. Air contains the following gases:
- nitrogen (78.08%);
- oxygen (20.94%);
- argon (0.93%);
- water vapor (0.40%);
- carbon dioxide (0.035%).
In addition to the gases listed above, the air also contains minimal amounts of neon (0.0018%), helium (0.0005%), methane (0.00017%), krypton (0.00014%), hydrogen (0.00005%), ammonia (0.0003%).
It is interesting to note thatYou can separate these components if you condense air, that is, turn it into a liquid state by increasing pressure and decreasing temperature. Since each component of the air has its own condensation temperature, in this way it is possible to isolate all components from the air, which is used in practice.
Air weight and factors that affect it

What prevents you from answering exactly the question, how much does a cubic meter of air weigh? Of course, a number of factors that can greatly influence this weight.
First, it's the chemical composition. Above are the data for the composition of clean air, however, at present this air is heavily polluted in many places on the planet, respectively, its composition will be different. So, near big cities, the air contains more carbon dioxide, ammonia, methane than the air in rural areas.
Secondly, humidity, that is, the amount of water vapor contained in the atmosphere. The more humid the air, the less it weighs, other things being equal.
Third, temperature. This is one of the important factors, the lower its value, the higher the air density, and, accordingly, the greater its weight.
Fourthly, atmospheric pressure, which directly reflects the number of air molecules in a certain volume, that is, its weight.
To understand how the combination of these factors affects the weight of air, let's take a simple example: the mass of one meter of dry cubic air at a temperature of 25 ° C, located near the surface of the earth,is 1.205 kg, but if we consider a similar volume of air near the sea surface at a temperature of 0 ° C, then its mass will already be equal to 1.293 kg, that is, it will increase by 7.3%.
Change in air density with altitude
As the altitude increases, the air pressure decreases, respectively, its density and weight decrease. Atmospheric air at pressures that are observed on Earth can be considered as an ideal gas in the first approximation. This means that the pressure and density of air are mathematically related to each other through the equation of state of an ideal gas: P=ρRT/M, where P is pressure, ρ is density, T is temperature in kelvins, M is the molar mass of air, R is the universal gas constant.
From the above formula, you can get the formula for the dependence of air density on height, given that the pressure changes according to the law P=P0+ρgh, where P 0 - pressure at the earth's surface, g - free fall acceleration, h - height. Substituting this formula for pressure into the previous expression, and expressing the density, we get: ρ(h)=P0M/(RT(h)+g(h)M h). Using this expression, you can determine the density of air at any height. Accordingly, the weight of air (more correctly, mass) is determined by the formula m(h)=ρ(h)V, where V is the given volume.
In the expression of the dependence of density on height, one can notice that the temperature and acceleration of free fall also depend on height. The last dependence can be neglected if we are talking about heights of no more than 1-2 km. As regards temperature, it altitude dependence is well described by the following empirical expression: T(h)=T0-0, 65h, where T0 is the air temperature near ground surface.
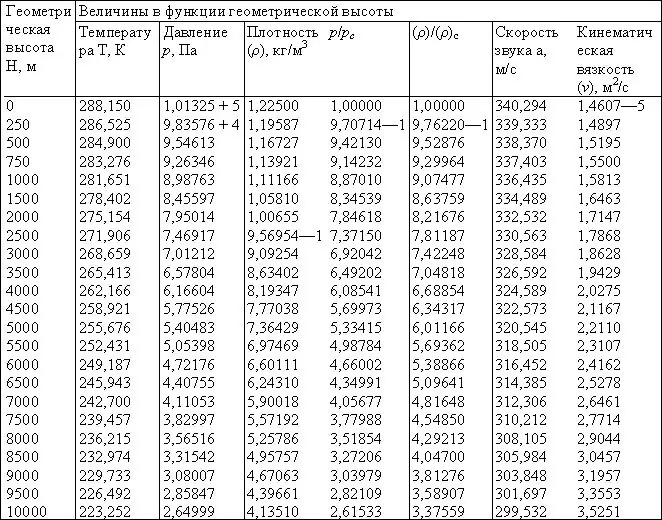
In order not to constantly calculate the density for each height, below is a table of the dependence of the main air characteristics on height (up to 10 km).
Which air is the heaviest
Having considered the main factors that determine the answer to the question of how much air weighs, you can understand which air will be the heaviest. In short, cold air always weighs more than warm air, since the density of the latter is lower, and dry air weighs more than moist air. The last statement is easy to understand, since the molar mass of air is 29 g/mol, and the molar mass of a water molecule is 18 g/mol, that is, 1.6 times less.
Determining the weight of air under given conditions

Now let's solve a specific problem. Let's answer the question of how much air weighs, occupying a volume of 150 liters, at a temperature of 288 K. Consider that 1 liter is a thousandth of a cubic meter, that is, 1 liter=0.001 m3. As for the temperature of 288 K, it corresponds to 15°C, that is, it is typical for many regions of our planet. The next step is to determine the density of the air. There are two ways to do this:
- Calculate using the above formula for a height of 0 meters above sea level. In this case, the value ρ=1.227 kg/m is obtained3
- Look at the table above, which is based on T0=288.15 K. The table contains the value ρ=1.225 kg/m 3.
Thus, we got two numbers that are in good agreement with each other. A slight difference is due to an error of 0.15 K in determining the temperature, and also to the fact that air is still not an ideal, but a real gas. Therefore, for further calculations, we take the average of the two obtained values, that is, ρ=1, 226 kg/m3.
Now, using the formula for the relationship between mass, density and volume, we get: m=ρV=1.226 kg/m30.150 m 3=0.1839 kg or 183.9 grams.
You can also answer how much a liter of air weighs under given conditions: m=1.226 kg/m30.001 m3=0.001226 kg or approximately 1.2 grams.
Why don't we feel the air pressing down on us

How much does 1 m3 of air weigh? A little over 1 kilogram. The entire atmospheric table of our planet puts pressure on a person with its weight of 200 kg! This is a large enough mass of air that could cause a lot of trouble to a person. Why don't we feel it? This is due to two reasons: firstly, there is also internal pressure inside the person himself, which counteracts external atmospheric pressure, and secondly, air, being a gas, exerts pressure in all directions equally, that is, pressures in all directions balance each other.






