Such an amazing and familiar square. It is symmetrical about its center and axes drawn along the diagonals and through the centers of the sides. And to look for the area of a square or its volume is not at all difficult. Especially if the length of its side is known.
A few words about the figure and its properties
The first two properties are related to the definition. All sides of the figure are equal to each other. After all, a square is a regular quadrilateral. Moreover, it must have all sides equal and the angles have the same value, namely, 90 degrees. This is the second property.
The third is related to the length of the diagonals. They also turn out to be equal to each other. Moreover, they intersect at right angles and at the midpoints.
Formula using only side length
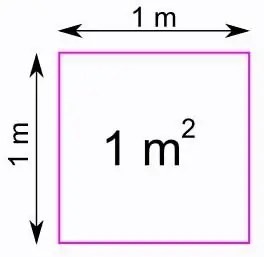
First, about the notation. For the length of the side, it is customary to choose the letter "a". Then the square area is calculated by the formula: S=a2.
It is easily obtained from the one known for the rectangle. In it, the length and width are multiplied. For a square, these two elements are equal. Therefore, in the formulathe square of this one value appears.
Formula in which the length of the diagonal appears
It is the hypotenuse in a triangle whose legs are the sides of the figure. Therefore, you can use the formula of the Pythagorean theorem and derive an equality in which the side is expressed through the diagonal.
After such simple transformations, we get that the square area through the diagonal is calculated by the following formula:
S=d2 / 2. Here the letter d denotes the diagonal of the square.
Perimeter formula
In such a situation, it is necessary to express the side through the perimeter and substitute it into the area formula. Since the figure has four identical sides, the perimeter will have to be divided by 4. This will be the value of the side, which can then be substituted into the initial one and calculate the area of the square.
The general formula looks like this: S=(Р/4)2.
Problems for calculations
1. There is a square. The sum of its two sides is 12 cm. Calculate the area of the square and its perimeter.
Decision. Since the sum of two sides is given, we need to find the length of one. Since they are the same, the known number must simply be divided by two. That is, the side of this figure is 6 cm.
Then its perimeter and area are easily calculated using the above formulas. The first is 24cm and the second is 36cm2.
Answer. The perimeter of a square is 24 cm and its area is 36 cm2.

2. Find the area of a square with a perimeter of 32 mm.
Decision. It is enough just to substitute the value of the perimeter in the formula written above. Although you can first find out the side of the square, and only then its area.
In both cases, the actions will first include division, and then exponentiation. Simple calculations lead to the fact that the area of the square represented is 64 mm2.
Answer. The desired area is 64 mm2.
3. The side of the square is 4 dm. Rectangle sizes: 2 and 6 dm. Which of the two figures has the larger area? How much?
Decision. Let the side of the square be marked with the letter a1, then the length and width of the rectangle are a2 and 2. To determine the area of a square, the value of a1 is supposed to be squared, and the value of a rectangle is to be multiplied by a2 and 2 . It's easy.
It turns out that the area of a square is 16 dm2, and a rectangle is 12 dm2. Obviously, the first figure is larger than the second. This is despite the fact that they are equal, that is, they have the same perimeter. To check, you can count the perimeters. At the square, the side must be multiplied by 4, you get 16 dm. Add the sides of the rectangle and multiply by 2. It will be the same number.
In the problem, you also need to answer how much the areas differ. To do this, subtract the smaller number from the larger number. The difference turns out to be 4 dm2.
Answer. The areas are 16 dm2 and 12 dm2. The square has 4 dm more2.
Proof problem
Condition. A square is built on the leg of an isosceles right triangle. An altitude is built to its hypotenuse, on which another square is built. Prove that the area of the first is twice that of the second.
Decision. Let us introduce notation. Let the leg be equal to a, and the height drawn to the hypotenuse be x. The area of the first square is S1, the second square is S2.
The area of the square built on the leg is easy to calculate. It turns out to be equal to a2. With the second value, things are not so simple.
First you need to find out the length of the hypotenuse. For this, the formula of the Pythagorean theorem is useful. Simple transformations lead to this expression: a√2.
Since the height in an isosceles triangle drawn to the base is also the median and height, it divides the large triangle into two equal isosceles right triangles. Therefore, the height is half the hypotenuse. That is, x \u003d (a √ 2) / 2. From here it is easy to find out the area S2. It turns out to be equal to a2/2.
Obviously, the recorded values differ exactly by a factor of two. And the second one is much less. As required to prove.
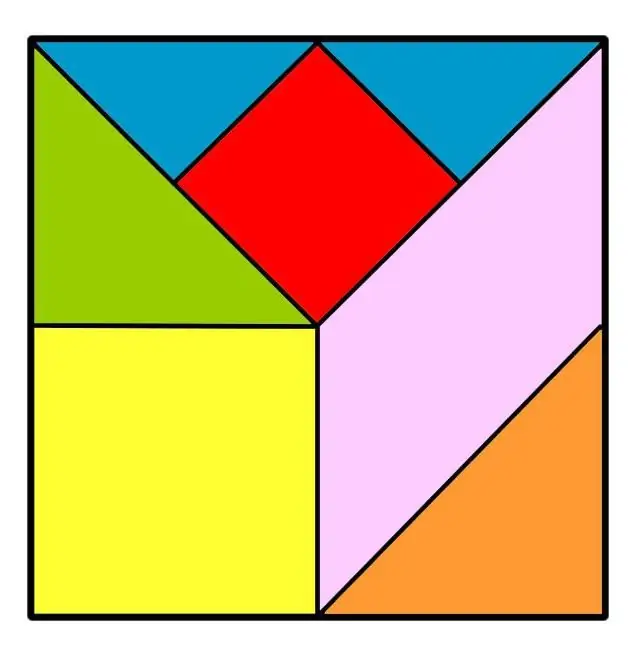
Unusual puzzle - tangram
It is made from a square. It must be cut into various shapes according to certain rules. Total parts should be 7.
The rules assume that during the game all the resulting parts will be used. Of these, you need to make other geometric shapes. For example,rectangle, trapezoid or parallelogram.
But it's even more interesting when the pieces turn into silhouettes of animals or objects. Moreover, it turns out that the area of all derivative figures is equal to that of the initial square.






