What is the axis of symmetry? This is a set of points that form a straight line, which is the basis of symmetry, that is, if a certain distance is set aside from the straight line on one side, then it will be reflected in the other direction in the same size. Anything can act as an axis - a point, a line, a plane, and so on. But it is better to talk about this with illustrative examples.
Symmetry
In order to understand what an axis of symmetry is, you need to delve into the very definition of symmetry. This is the correspondence of a certain fragment of the body with respect to any axis, when its structure is unchanged, and the properties and shape of such an object remain the same with respect to its transformations. We can say that symmetry is the property of bodies to display. When a fragment cannot have such a match, it is called asymmetry or arrhythmia.
Some shapes are not symmetrical, that's why they are called irregular or asymmetric. These include various trapezoids (except isosceles), triangles (except isosceles and equilateral) and others.
Types of symmetry
We will also discuss some types of symmetry in order to fully explore this concept. They are divided like this:
- Axial. The axis of symmetry is a straight line passing through the center of the body. Like this? If you impose parts around the axis of symmetry, then they will be equal. This can be seen in the example of a sphere.
- Mirror. The axis of symmetry here is a straight line, relative to which the body can be reflected and inversely displayed. For example, butterfly wings are mirror-symmetrical.
- Central. The axis of symmetry is the point in the center of the body, relative to which, under all transformations, the parts of the body are equal when superimposed.
History of symmetry
The very concept of symmetry is often the starting point in the theories and hypotheses of ancient scientists who were confident in the mathematical harmony of the universe, as well as in the manifestation of the divine principle. The ancient Greeks firmly believed that the universe is symmetrical, because symmetry is magnificent. Man has long used the idea of symmetry in his knowledge of the picture of the universe.
In the 5th century BC, Pythagoras considered the sphere the most perfect form and thought that the Earth has the shape of a sphere and moves in the same way. He also believed that the Earth moves in the form of some kind of "central fire", around which 6 planets (known at that time), the Moon, the Sun and all other stars should have revolved.
And the philosopher Plato considered polyhedrons to be the personification of the four natural elements:
- tetrahedron is fire, as its toppointing up;
- cube - earth, as it is the most stable body;
- octahedron - air, no explanation;
- icosahedron - water, since the body does not have rough geometric shapes, angles, and so on;
- the image of the entire universe was the dodecahedron.
Because of all these theories, regular polyhedra are called Platonic solids.
Symmetry was used by the architects of Ancient Greece. All their buildings were symmetrical, as evidenced by the images of the ancient temple of Zeus at Olympia.

Dutch artist M. C. Escher also used symmetry in his paintings. In particular, a mosaic of two birds flying towards became the basis of the painting "Day and Night".
Also, our art critics did not neglect the rules of symmetry, as can be seen in the example of the painting by V. M. Vasnetsov "Heroes".
What can I say, symmetry has been a key concept for all artists for many centuries, but in the 20th century its meaning was also appreciated by all figures of the exact sciences. The exact evidence is physical and cosmological theories, for example, the theory of relativity, string theory, absolutely all of quantum mechanics. From the time of Ancient Babylon and ending with the cutting-edge discoveries of modern science, the paths of studying symmetry and discovering its basic laws can be traced.
Symmetry of geometric shapes and bodies
Let's take a closer look at geometric bodies. For example, the axis of symmetry of a parabola is a straight line passing through its vertex and cutting through the given bodyin half. This figure has one single axis.
But with geometric shapes, the situation is different. The axis of symmetry of the rectangle is also straight, but there are several of them. You can draw an axis parallel to the width segments, or you can draw the length. But not everything is so simple. Here the line has no axes of symmetry, since its end is not defined. Only central symmetry could exist, but, accordingly, there will not be one either.
You should also know that some bodies have many axes of symmetry. This is easy to guess. You don't even need to talk about how many axes of symmetry a circle has. Any line passing through the center of the circle is such, and there are an infinite number of these lines.
Some quadrilaterals can have two axes of symmetry. But the second must be perpendicular. This happens in the case of the rhombus and the rectangle. In the first axis of symmetry - the diagonals, and in the second - the middle lines. The set of such axes is only for the square.
Symmetry in nature
Nature amazes with many examples of symmetry. Even our human body is symmetrical. Two eyes, two ears, nose and mouth are located symmetrically about the central axis of the face. Arms, legs and the whole body in general are arranged symmetrically to the axis passing through the middle of our body.
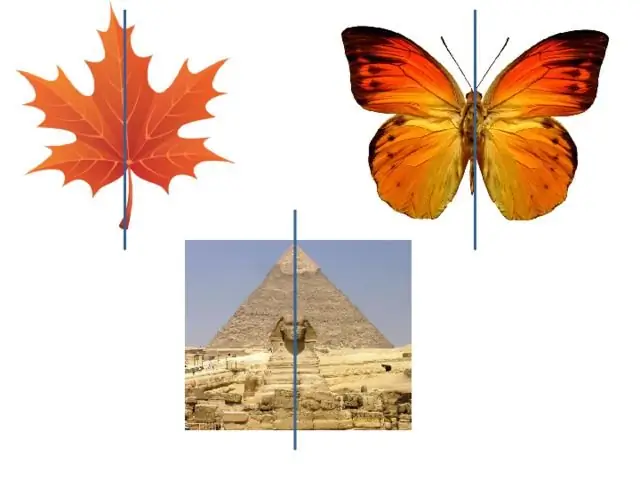
And how many examples surround us all the time! These are flowers, leaves, petals, vegetables and fruits, animals and even honeycombs of bees have a pronounced geometric shape and symmetry. All naturearranged in an orderly manner, everything has its place, which once again confirms the perfection of the laws of nature, in which symmetry is the main condition.
Conclusion
We are constantly surrounded by some phenomena and objects, such as a rainbow, a drop, flowers, petals, and so on. Their symmetry is obvious, to some extent it is due to gravity. Often in nature, the concept of "symmetry" is understood as a regular change of day and night, seasons, and so on.

Similar properties are observed wherever there is order and equality. Also, the very laws of nature - astronomical, chemical, biological and even genetic - are subject to certain principles of symmetry, as they have a perfect system, which means that the balance has an all-encompassing scale. Therefore, axial symmetry is one of the fundamental laws of the universe as a whole.






