In order to be able to depict on paper objects that in reality do not have very “convenient” sizes, people came up with a scale. In fact, this explains what the scale is for.
When the school curriculum begins to reveal the concept of scale
For the first time, children come across this word while studying maps and plans of the area. The teacher explains why the scale is needed, what it shows, using atlases as an example. It is explained that any geographic feature is so large that it would be difficult and inconvenient to depict it in full size.
People drew the terrain in a reduced form, but for this they did not use any exact ratios. Now it is being done smarter - each dash and line depicted on the map has a size that can be multiplied by a certain number and find out the true length and width.
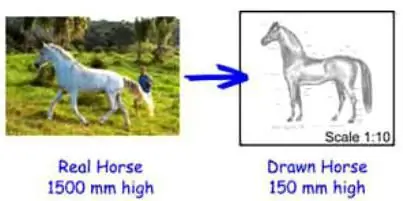
Record scale: the first way to read
The scale is indicated by two numbers separated by a colon. The first digit indicates the units of dimension in the figure, the secondindicates how many real units in the figure corresponds to the first number. For example, if a scale of 1:1000 is indicated on some plan, and the units of dimensions are indicated in centimeters, then one centimeter in the figure corresponds to 1000 cm in reality. So what is the scale for? With its help, you can not only reduce certain objects on the graphic plan, but also accurately calculate their real size.
The second way to record the scale: what is convenient?
The previous method of writing the scale through a colon is called numerical. But there is also a named scale. His record is as follows: 1 cm - 20 km. It turns out that in this way it is possible to compactly write down huge scales that will not be expressed by numbers with several zeros, if a situation has occurred when it is necessary to indicate several hundred kilometers in one centimeter. At the same time, it is immediately clear how much, what and in what. This record is more intuitive and clear.
Scaling in drawing: what complements the previously studied concept
The concept of scale is encountered not only in geography, but also in the study of such a subject as drawing. The same principles are used to depict various objects. But there is a significant difference: here the concept of what scale is for is also expanded by the fact that it can be used to depict small details on a larger scale. In geography, this is not discussed, because there are no such small objects in geography that there is a need to enlarge them. Continents and mountains, rivers and lakes are in any case larger than sheets of A4 paper or evenA1.
Studying drawing, you can use the scale to depict in a larger form the smallest details, such as a bolt or cog.
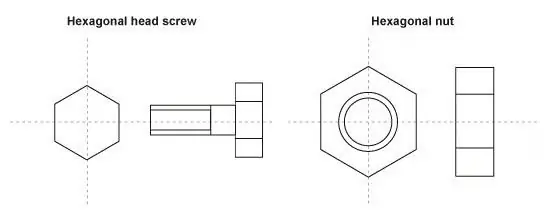
So, why do we need a scale in this case? With its help, you can more conveniently, clearly and in detail depict a small element. In this case, the reverse occurs in the notation: the first number will be greater than the second, and the notation 100:1 will sound something like this: 100 metric units of the image corresponds to one unit of the actual size.
A few clarifying examples
What is the scale for, what does it show in the case of an image of a smaller object, which is the result on paper? Again, we have the exact ratio of the dimensions of the image of the part and the real object. Recall the same scale of 100:1. It turns out that in a hundred millimeters in the figure there is only one millimeter of real size. If a part is 500 millimeters wide in the picture, its actual width is only 5 millimeters.
If we recall the first case, the image on a sheet of a smaller copy of a large object, the scale of 1:100 will mean that one millimeter in the picture contains 100 millimeters of the true size. So, if an object is 80 millimeters long on a drawing or map, the actual length of the object will be 8000 millimeters. A clear example of what scale is for and what a convenient invention of mankind.
The main thing in using the scale is immediatelyremember that the first number refers to the image and the second to the actual size of the objects. In order not to be confused in the future, in order to consolidate these foundations, practical classes in geography are held in schools so that children explain and calculate the sizes of real objects several times using the atlas. The same thing happens in drawing lessons.
Summarize
What is the scale for? The answer to this question consists of three points that you just need to remember:
- First - the scale is necessary to display large objects on a surface that is convenient for viewing.
- Second - the scale is needed in order to depict small objects in a larger size.
- Third - the scale is needed in order to be able to accurately determine the size of a real object, regardless of its initial size, small or large.






