When studying the properties of a quadratic equation, a restriction was set - for a discriminant less than zero, there is no solution. It was immediately stipulated that we are talking about a set of real numbers. The inquisitive mind of a mathematician will be interested - what is the secret contained in the clause about real values?
Over time, mathematicians introduced the concept of complex numbers, where the unit is taken as the conditional value of the root of the second degree of minus one.
Historical background
Mathematical theory develops sequentially, from simple to complex. Let's figure out how the concept called "complex number" arose and why it is needed.
From time immemorial, the basis of mathematics was the usual account. The researchers knew only the natural set of values. Addition and subtraction were simple. As economic relations became more complex, multiplication began to be used instead of adding the same values. There is a reverse operation tomultiplication - division.
The concept of a natural number limited the use of arithmetic operations. It is impossible to solve all division problems on the set of integer values. Working with fractions led first to the concept of rational values, and then to irrational values. If for the rational one can indicate the exact location of a point on the line, then for the irrational such a point cannot be specified. You can only approximate the interval. The union of rational and irrational numbers formed a real set, which can be represented as a certain line with a given scale. Each step along the line is a natural number, and between them are rational and irrational values.
The era of theoretical mathematics has begun. The development of astronomy, mechanics, physics required the solution of more and more complex equations. In general, the roots of the quadratic equation were found. When solving a more complex cubic polynomial, scientists ran into a contradiction. The concept of a cube root from a negative makes sense, but for a square root, uncertainty is obtained. Moreover, the quadratic equation is only a special case of the cubic one.
In 1545, the Italian J. Cardano proposed to introduce the concept of an imaginary number.

This number is the second root of minus one. The term complex number was finally formed only three hundred years later, in the works of the famous mathematician Gauss. He proposed formally extending all the laws of algebra to the imaginary number. The real line has been extended toplanes. The world is bigger.
Basic concepts
Recall a number of functions that have restrictions on the real set:
- y=arcsin(x), defined between negative and positive 1.
- y=ln(x), decimal logarithm makes sense with positive arguments.
- square root y=√x, calculated only for x ≧ 0.
Denoting i=√(-1), we introduce such a concept as an imaginary number, this will remove all restrictions from the domain of definition of the above functions. Expressions like y=arcsin(2), y=ln(-4), y=√(-5) make sense in some space of complex numbers.
The algebraic form can be written as an expression z=x + i×y on the set of real x and y values, and i2 =-1.
The new concept removes all restrictions on the use of any algebraic function and resembles a graph of a straight line in coordinates of real and imaginary values.
Complex plane
The geometric form of complex numbers visually allows us to represent many of their properties. On the Re(z) axis we mark the real x values, on the Im(z) - the imaginary values of y, then the z point on the plane will display the required complex value.
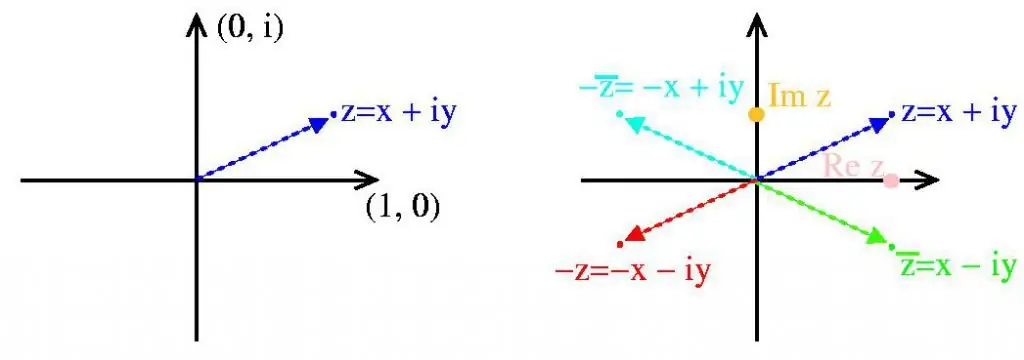
Definitions:
- Re(z) - real axis.
- Im(z) - means the imaginary axis.
- z - conditional point of a complex number.
- The numerical value of the length of the vector from zero to z is calledmodule.
- Real and imaginary axes divide the plane into quarters. With a positive value of the coordinates - I quarter. When the argument of the real axis is less than 0, and the imaginary axis is greater than 0 - II quarter. When the coordinates are negative - III quarter. The last, fourth quarter contains many positive real values and negative imaginary values.
Thus, on a plane with x and y coordinate values, one can always visualize a point of a complex number. The character i is introduced to separate the real part from the imaginary one.
Properties
- When the value of the imaginary argument is zero, we get just a number (z=x), which is located on the real axis and belongs to the real set.
- Special case when the value of the real argument becomes zero, the expression z=i×y corresponds to the location of the point on the imaginary axis.
- The general form of z=x + i×y will be for non-zero values of the arguments. Indicates the location of the point characterizing the complex number in one of the quarters.
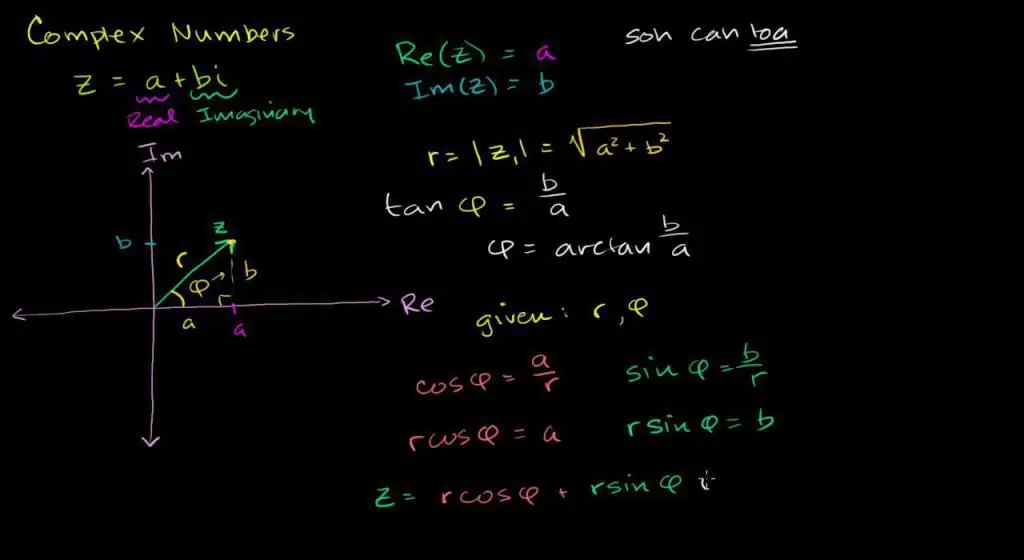
Trigonometric notation
Recall the polar coordinate system and the definition of the trigonometric functions sin and cos. It is obvious that with the help of these functions it is possible to describe the location of any point on the plane. To do this, it is enough to know the length of the polar beam and the angle of inclination to the real axis.
Definition. An entry of the form ∣z ∣ multiplied by the sum of the trigonometric functions cos(ϴ) and the imaginary part i ×sin(ϴ) is called a trigonometric complex number. Here the designation is the angle of inclination to the real axis
ϴ=arg(z) and r=∣z∣, beam length.
From the definition and properties of trigonometric functions, a very important Moivre formula follows:
zn =r × (cos(n × ϴ) + i × sin(n × ϴ)).
Using this formula, it is convenient to solve many systems of equations containing trigonometric functions. Especially when the problem of raising to a power arises.
Module and phase
To complete the description of a complex set, we propose two important definitions.
Knowing the Pythagorean theorem, it is easy to calculate the length of the beam in the polar coordinate system.
r=∣z∣=√(x2 + y2), such a notation on a complex space is called a "module" and characterizes the distance from 0 to a point on the plane.
The angle of inclination of the complex beam to the real line ϴ is commonly called the phase.
The definition shows that the real and imaginary parts are described using cyclic functions. Namely:
- x=r × cos(ϴ);
- y=r × sin(ϴ);
Reversely, the phase is related to algebraic values through the formula:
ϴ=arctan(x / y) + µ, correction µ is introduced to take into account the periodicity of geometric functions.
Euler formula
Mathematicians often use the exponential form. Complex plane numbers are written as expressions
z=r × ei×ϴ , which follows from the Euler formula.

This record is widely used for the practical calculation of physical quantities. Form of presentation in the formexponential complex numbers is especially convenient for engineering calculations, where it becomes necessary to calculate circuits with sinusoidal currents and it is necessary to know the value of integrals of functions with a given period. The calculations themselves serve as a tool in the design of various machines and mechanisms.
Define operations
As already noted, all algebraic laws of working with basic mathematical functions apply to complex numbers.
Sum operation
When adding complex values, their real and imaginary parts are also added.
z=z1 + z2 where z1 and z 2 - general complex numbers. Transforming the expression, after opening the brackets and simplifying the notation, we get the real argument x=(x1 + x2), the imaginary argument y=(y 1 + y2).
On the graph, it looks like the addition of two vectors, according to the well-known parallelogram rule.
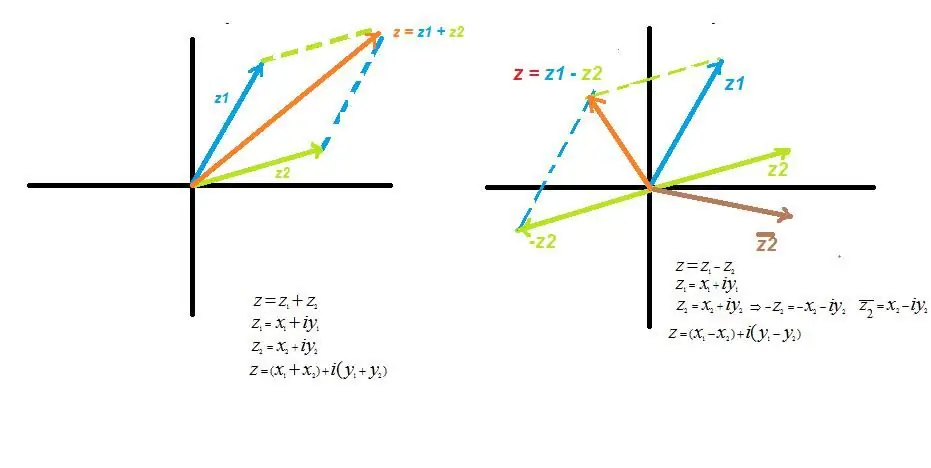
Subtraction operation
Considered as a special case of addition, when one number is positive, the other is negative, that is, located in the mirror quarter. Algebraic notation looks like the difference between real and imaginary parts.
z=z1 - z2, or, taking into account the values of the arguments, similarly to the addition operation, we obtain for the real values x=(x1 - x2) and imaginary y=(y1 - y2).
Multiplication on the complex plane
Using the rules for working with polynomials, we derive the formulato solve complex numbers.
Following the general algebraic rules z=z1×z2, describe each argument and list similar ones. The real and imaginary parts can be written like this:
- x=x1 × x2 - y1 × y 2,
- y=x1 × y2 + x2 × y 1.
It looks more beautiful if we use exponential complex numbers.
The expression looks like this: z=z1 × z2 =r1 × eiϴ1 × r2 × e iϴ2=r1 × r 2 × ei(ϴ1+ϴ 2).
Further simply, the modules are multiplied and the phases are added.
Division
When considering the operation of division as the inverse of multiplication, we obtain a simple expression in exponential notation. Dividing the value z1 by z2 is the result of dividing their modules and phase difference. Formally, when using the exponential form of complex numbers, it looks like this:
z=z1 / z2 =r1 × e iϴ1 / r2 × ei ϴ2=r1 / r2× ei(ϴ1-ϴ 2).
In the form of algebraic notation, the operation of dividing the numbers of the complex plane is written a little more complicated:
z=z1 / z2.
Describing arguments and performing polynomial transformations, it is easy to get valuesx=x1 × x2 + y1 × y2, respectively y=x2 × y1 - x1 × y2 , however, within the described space, this expression makes sense if z2 ≠ 0.
Extract the root
All of the above can be applied when defining more complex algebraic functions - raising to any power and inverse to it - extracting the root.
Using the general concept of raising to the power n, we get the definition:
zn =(r × eiϴ).
Using common properties, rewrite as:
zn =rn × eiϴ.
We got a simple formula for raising a complex number to a power.
From the definition of the degree we get a very important consequence. An even power of the imaginary unit is always 1. Any odd power of the imaginary unit is always -1.
Now let's study the inverse function - extracting the root.
For ease of notation, let's take n=2. The square root w of the complex value z on the complex plane C is considered to be the expression z=±, valid for any real argument greater than or equal to zero. For w ≦ 0, there is no solution.
Let's look at the simplest quadratic equation z2 =1. Using complex number formulas, rewrite r2 × e i2ϴ =r2 × ei2ϴ=ei0. It can be seen from the record that r2 =1 and ϴ=0, therefore, we have the only solution equal to 1. But this contradicts the notion that z=-1 also fits the definition of a square root.
Let's figure out what we don't take into account. If we recall the trigonometric notation, then we restore the statement - with a periodic change in the phase ϴ, the complex number does not change. Let p denote the value of the period, then we have r2 × ei2ϴ =e i(0+p), whence 2ϴ=0 + p, or ϴ=p / 2. Therefore, ei0 =1 and ei p/2 =-1. We got the second solution, which corresponds to the general understanding of the square root.
So, to find an arbitrary root of a complex number, we will follow the procedure.
- Write the exponential form w=∣w∣ × ei(arg(w) + pk), k is an arbitrary integer.
- The desired number is also represented in the Euler form z=r × eiϴ.
- Use the general definition of the root extraction function r ei ϴ =∣w∣ × ei(arg ( w) + pk).
- From the general properties of the equality of modules and arguments, we write rn =∣w∣ and nϴ=arg (w) + p×k.
- The final record of the root of a complex number is described by the formula z=√∣w∣ × ei (arg (w) + pk ) / .
- Note. The value of ∣w∣, by definition,is a positive real number, so the root of any degree makes sense.
Field and conjugation
In conclusion, we give two important definitions that are of little importance for solving applied problems with complex numbers, but are essential for the further development of mathematical theory.
The expressions for addition and multiplication are said to form a field if they satisfy the axioms for any elements of the complex plane z:
- The complex sum does not change from changing places of complex terms.
- The statement is true - in a complex expression, any sum of two numbers can be replaced by their value.
- There is a neutral value 0 for which z + 0=0 + z=z is true.
- For any z there is an opposite - z, addition to which gives zero.
- When changing places of complex factors, the complex product does not change.
- The multiplication of any two numbers can be replaced by their value.
- There is a neutral value 1, multiplication by which does not change the complex number.
- For every z ≠ 0, there is an inverse of z-1, which multiplies by 1.
- Multiplying the sum of two numbers by a third is equivalent to the operation of multiplying each of them by this number and adding the results.
- 0 ≠ 1.
The numbers z1 =x + i×y and z2 =x - i×y are called conjugate.
Theorem. For conjugation, the statement is true:
- The conjugation of the sum is equal to the sum of conjugate elements.
- The conjugate of the product isproduct of conjugations.
- The conjugation of conjugation is equal to the number itself.
In general algebra, such properties are called field automorphisms.
Examples
Following the given rules and formulas of complex numbers, you can easily operate with them.
Let's consider the simplest examples.
Problem 1. Using the equation 3y +5 x i=15 - 7i, determine x and y.
Decision. Recall the definition of complex equalities, then 3y=15, 5x=-7. Therefore, x=-7 / 5, y=5.
Task 2. Calculate the values 2 + i28 and 1 + i135.
Decision. Obviously, 28 is an even number, from the consequence of the definition of a complex number in the power we have i28 =1, which means that the expression 2 + i28 =3. The second value, i135 =-1, then 1 + i135 =0.
Task 3. Calculate the product of the values 2 + 5i and 4 + 3i.
Decision. From the general properties of multiplication of complex numbers, we obtain (2 + 5i)X(4 + 3i)=8 - 15 + i(6 + 20). The new value will be -7 + 26i.
Task 4. Calculate the roots of the equation z3 =-i.
Decision. There are several ways to find a complex number. Let's consider one of the possible. By definition, ∣ - i∣=1, the phase for -i is -p / 4. The original equation can be rewritten as r3ei 3ϴ =e-p/4+pk, from where z=e-p / 12 + pk/3, for any integer k.
The solution set has the form (e-ip/12,eip/4, ei2 p/3).
Why do we need complex numbers
History knows many examples when scientists, working on a theory, do not even think about the practical application of their results. Mathematics is, first of all, a game of the mind, a strict adherence to cause-and-effect relationships. Almost all mathematical constructions are reduced to solving integral and differential equations, and those, in turn, with some approximation, are solved by finding the roots of polynomials. Here we first encounter the paradox of imaginary numbers.
Scientists naturalists, solving completely practical problems, resorting to solutions of various equations, discover mathematical paradoxes. The interpretation of these paradoxes leads to absolutely amazing discoveries. The dual nature of electromagnetic waves is one such example. Complex numbers play a crucial role in understanding their properties.
This, in turn, has found practical application in optics, radio electronics, energy and many other technological fields. Another example, much more difficult to understand physical phenomena. Antimatter was predicted at the tip of a pen. And only many years later, attempts to physically synthesize it begin.
Don't think that only in physics there are such situations. No less interesting discoveries are made in wildlife, in the synthesis of macromolecules, during the study of artificial intelligence. And it's all thanks toexpansion of our consciousness, moving away from simple addition and subtraction of natural values.






