Many problems in physics can be successfully solved if the laws of conservation of one or another quantity during the considered physical process are known. In this article, we will consider the question of what is the momentum of the body. And we will also carefully study the law of conservation of momentum.
General concept
More correctly, it's about the amount of movement. The patterns associated with it were first studied by Galileo at the beginning of the 17th century. Based on his writings, Newton published a scientific paper during this period. In it, he clearly and clearly outlined the basic laws of classical mechanics. Both scientists understood the quantity of motion as a characteristic, which is expressed by the following equality:
p=mv.
Based on it, the value p determines both the inertial properties of a body with mass m and its kinetic energy, which depends on the speed v.
The momentum is called the amount of motion because its change is connected with the momentum of the force through Newton's second law. It's not hard to show it. You only need to find the derivative of the momentum with respect to time:
dp/dt=mdv/dt=ma=F.
From where we get:
dp=Fdt.
The right side of the equation is called the momentum of the force. It shows the amount of change in momentum over time dt.

Closed systems and internal forces
Now we have to deal with two more definitions: what is a closed system, and what are the internal forces. Let's consider in more detail. Since we are talking about mechanical movement, then a closed system is understood as a set of objects that are not affected by external bodies in any way. That is, in such a structure, the total energy and the total amount of matter are conserved.
The concept of internal forces is closely related to the concept of a closed system. Under those, only those interactions are considered that are realized exclusively between the objects of the structure under consideration. That is, the action of external forces is completely excluded. In the case of the motion of the bodies of the system, the main types of interaction are mechanical collisions between them.
Determination of the law of conservation of body momentum
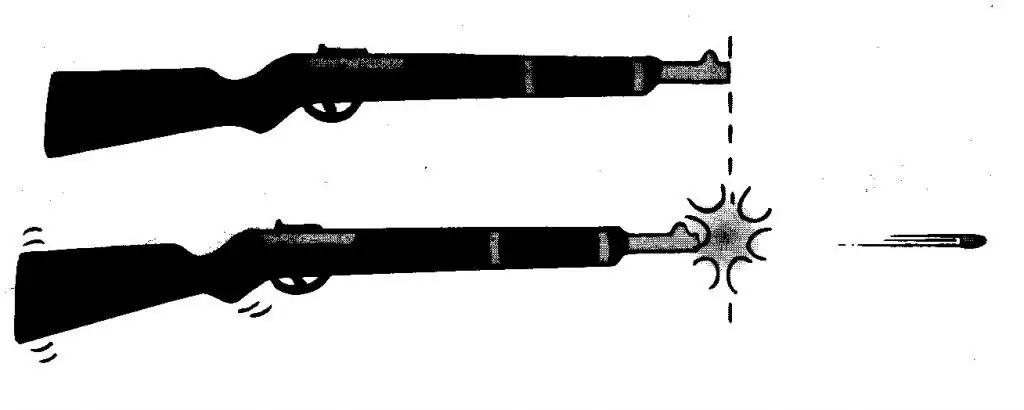
Momentum p in a closed system, in which only internal forces act, remains constant for an arbitrarily long time. It cannot be changed by any internal interactions between bodies. Since this quantity (p) is a vector, this statement should be applied to each of its three components. The formula for the law of conservation of body momentum can be written as follows:
px=const;
py=const;
pz=const.
This law is convenient to apply when solving practical problems in physics. In this case, the one-dimensional or two-dimensional case of the motion of bodies before their collision is often considered. It is this mechanical interaction that leads to a change in the momentum of each body, but their total momentum remains constant.
As you know, mechanical collisions can be absolutely inelastic and, conversely, elastic. In all these cases, the momentum is conserved, although in the first type of interaction, the kinetic energy of the system is lost as a result of its conversion into heat.
Example problem
After getting acquainted with the definitions of the momentum of the body and the law of conservation of momentum, we will solve the following problem.
It is known that two balls, each with a mass m=0.4 kg, roll in the same direction with speeds of 1 m/s and 2 m/s, while the second follows the first. After the second ball overtook the first, an absolutely inelastic collision of the considered bodies took place, as a result of which they began to move as a whole. It is necessary to determine the joint speed of their forward movement.

Solve this problem is not difficult if you apply the following formula:
mv1+ mv2=(m+m)u.
Here the left side of the equation represents the momentum before the balls collided, the right - after the collision. The speed u will be:
u=(mv1+mv2)/(2m)=(v1+ v2)/ 2;
u=1.5 m/s.
As you can see, the final result does not depend on the mass of the balls, since it is the same.
Note that if, according to the condition of the problem, the collision would be absolutely elastic, then to obtain an answer, one should use not only the law of conservation of the value of p, but also the law of conservation of the kinetic energy of the system of balls.
Body rotation and angular momentum
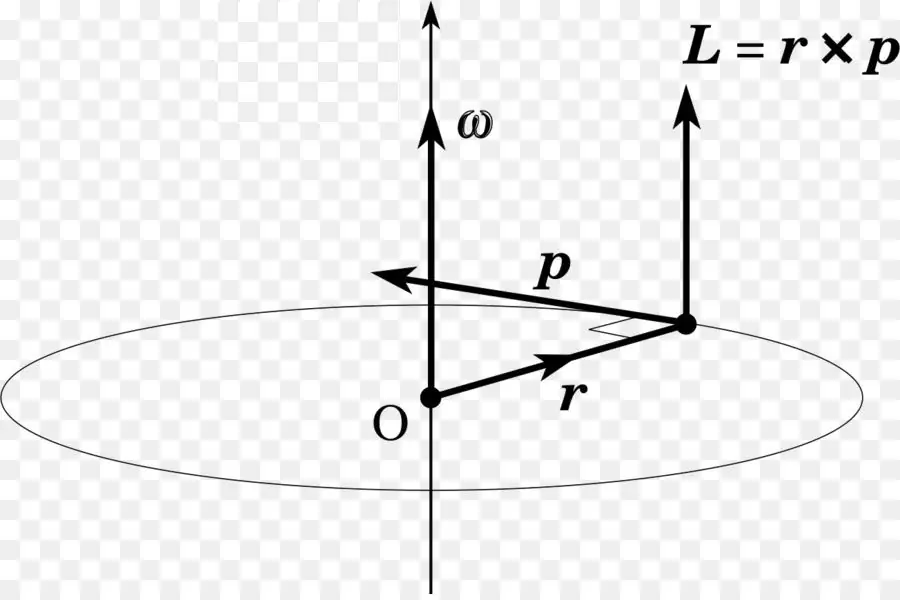
All that was said above refers to the translational movement of objects. The dynamics of rotational motion is in many ways similar to its dynamics with the difference that it uses the concepts of moments, for example, the moment of inertia, the moment of force and the moment of impulse. The latter is also called angular momentum. This value is determined by the following formula:
L=pr=mvr.
This equality says that to find the angular momentum of a material point, you should multiply its linear momentum p by the radius of rotation r.
Through the angular momentum, Newton's second law for the movement of rotation is written in this form:
dL=Mdt.
Here M is the moment of force, which during the time dt acts on the system, giving it an angular acceleration.
The law of conservation of angular momentum of the body
The last formula in the previous paragraph of the article says that a change in the value of L is possible only if some external forces act on the system, creating a non-zero torque M.in the absence of such, the value of L remains unchanged. The law of conservation of angular momentum states that no internal interactions and changes in the system can lead to a change in the module L.
If we use the concepts of momentum inertia I and angular velocity ω, then the conservation law under consideration will be written as:
L=Iω=const.

It manifests itself when, during the performance of a number with rotation in figure skating, an athlete changes the shape of his body (for example, presses his hands to the body), while changing his moment of inertia and inversely proportional to the angular velocity.
Also, this law is used to perform rotations around its own axis of artificial satellites during their orbital movement in outer space. In the article, we considered the concept of the momentum of a body and the law of conservation of the momentum of a system of bodies.






