Pyramid is a polyhedron based on a polygon. All faces, in turn, form triangles that converge at one vertex. Pyramids are triangular, quadrangular, and so on. In order to determine which pyramid is in front of you, it is enough to count the number of corners at its base. The definition of "height of the pyramid" is very often found in geometry problems in the school curriculum. In the article we will try to consider different ways to find it.
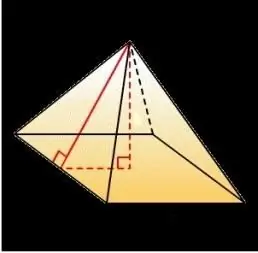
Parts of the pyramid
Each pyramid consists of the following elements:
- side faces that have three corners and converge at the top;
- apothem is the height that descends from its top;
- the top of the pyramid is a point that connects the side edges, but does not lie in the plane of the base;
- base is a polygon that does not contain a vertex;
- the height of the pyramid is a segment that intersects the top of the pyramid and forms a right angle with its base.
How to find the height of a pyramid if you know itvolume
Through the pyramid volume formula V=(Sh)/3 (in the formula V is the volume, S is the area of the base, h is the height of the pyramid) we find that h=(3V)/S. To consolidate the material, let's immediately solve the problem. In a triangular pyramid, the base area is 50 cm2, while its volume is 125 cm3. The height of the triangular pyramid is unknown, which we need to find. Everything is simple here: we insert the data into our formula. We get h=(3125)/50=7.5 cm.
How to find the height of a pyramid if the length of the diagonal and its edge are known
As we remember, the height of the pyramid forms a right angle with its base. And this means that the height, edge and half of the diagonal together form a right triangle. Many, of course, remember the Pythagorean theorem. Knowing two dimensions, it will not be difficult to find the third value. Recall the well-known theorem a²=b² + c², where a is the hypotenuse, and in our case, the edge of the pyramid; b - the first leg or half of the diagonal and c - respectively, the second leg, or the height of the pyramid. From this formula c²=a² - b².
Now the problem: in a regular pyramid, the diagonal is 20 cm, while the length of the edge is 30 cm. You need to find the height. Solve: c²=30² - 20²=900-400=500. Hence c=√ 500=about 22, 4.
How to find the height of a truncated pyramid
It is a polygon with a section parallel to its base. The height of a truncated pyramid is the segment that connects its two bases. The height can be found at the correct pyramid if they are knownthe lengths of the diagonals of both bases, as well as the edge of the pyramid. Let the diagonal of the larger base be d1, while the diagonal of the smaller base be d2, and the edge be of length l. To find the height, you can lower the heights from the two upper opposite points of the diagram to its base. We see that we have two right-angled triangles, it remains to find the lengths of their legs. To do this, subtract the smaller diagonal from the larger diagonal and divide by 2. So we will find one leg: a \u003d (d1-d2) / 2. After that, according to the Pythagorean theorem, we just have to find the second leg, which is the height of the pyramid.
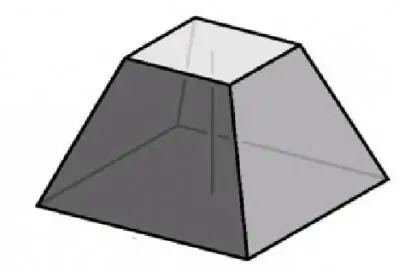
Now let's put the whole thing into practice. We have a task ahead of us. The truncated pyramid has a square at the base, the diagonal length of the larger base is 10 cm, while the smaller one is 6 cm, and the edge is 4 cm. It is required to find the height. To begin with, we find one leg: a \u003d (10-6) / 2 \u003d 2 cm. One leg is 2 cm, and the hypotenuse is 4 cm. It turns out that the second leg or height will be 16-4 \u003d 12, that is, h \u003d √12=about 3.5 cm.






