The phenomenon of refraction. Refractive index of air

2024 Author: Angel Austin | [email protected]. Last modified: 2024-01-02 17:39

Optics is one of the oldest branches of physics. Since ancient Greece, many philosophers have been interested in the laws of motion and propagation of light in various transparent materials such as water, glass, diamond and air. This article discusses the phenomenon of light refraction, focusing on the refractive index of air.

The effect of refraction of the light beam

Everyone in his life faced hundreds of times with the manifestation of this effect when he looked at the bottom of a reservoir or at a glass of water with some object placed in it. At the same time, the reservoir did not seem as deep as it actually was, and objects in a glass of water looked deformed or broken.

The phenomenon of refraction of a light beam is a break in its rectilinear trajectory when it crosses the interface between two transparent materials. Summarizing a large number of experimental data, at the beginning of the 17th century, the Dutchman Willebrord Snell received a mathematical expression,which accurately described this phenomenon. This expression is usually written in the following form:

₁sin(θ₁)=n₂sin(θ ₂)=const.

Here n₁, n₂ are the absolute refractive indices of light in the corresponding material, θ_{1 and θ}2 _{- the angles between the incident and refracted beams and the perpendicular to the interface plane, which is drawn through the point of intersection of the beam and this plane.}

This formula is called Snell's or Snell-Descartes' law (it was the Frenchman who wrote it down in the presented form, while the Dutchman used not sines, but units of length).

Besides this formula, the phenomenon of refraction is described by another law, which is geometric in nature. It lies in the fact that the marked perpendicular to the plane and two rays (refracted and incident) lie in the same plane.

Absolute refractive index

This value is included in the Snell formula, and its value plays an important role. Mathematically, the refractive index n corresponds to the formula:

n=c/v.

The symbol c is the speed of electromagnetic waves in vacuum. It is approximately 310⁸m/s. The value v is the speed of light in the medium. Thus, the refractive index reflects the amount of slowing down of light in a medium with respect to airless space.

There are two important implications from the formula above:

• value n is always greater than 1 (for vacuum it is equal to one);
• this is a dimensionless quantity.

For example, the refractive index of air is 1.00029, while for water it is 1.33.

The refractive index is not a constant value for a particular medium. It depends on the temperature. Moreover, for each frequency of an electromagnetic wave, it has its own meaning. So, the above figures correspond to a temperature of 20 ^oC and the yellow part of the visible spectrum (wavelength is about 580-590 nm).

The dependence of the value of n on the frequency of light is manifested in the decomposition of white light by a prism into a number of colors, as well as in the formation of a rainbow in the sky during heavy rain.

Refractive index of light in air

Its value has already been given above (1, 00029). Since the refractive index of air differs only in the fourth decimal place from zero, then for solving practical problems it can be considered equal to one. A small difference of n for air from unity indicates that light is practically not slowed down by air molecules, which is associated with its relatively low density. So, the average density of air is 1.225 kg/m³, that is, it is more than 800 times lighter than fresh water.

Air is an optically thin medium. The very process of slowing down the speed of light in a material is of a quantum nature and is associated with the acts of absorption and emission of photons by the atoms of matter.

Changes in the composition of the air (for example, an increase in the content of water vapor in it) and changes in temperature lead to significant changes in the indicatorrefraction. A striking example is the mirage effect in the desert, which occurs due to the difference in the refractive indices of air layers with different temperatures.

Glass-air interface

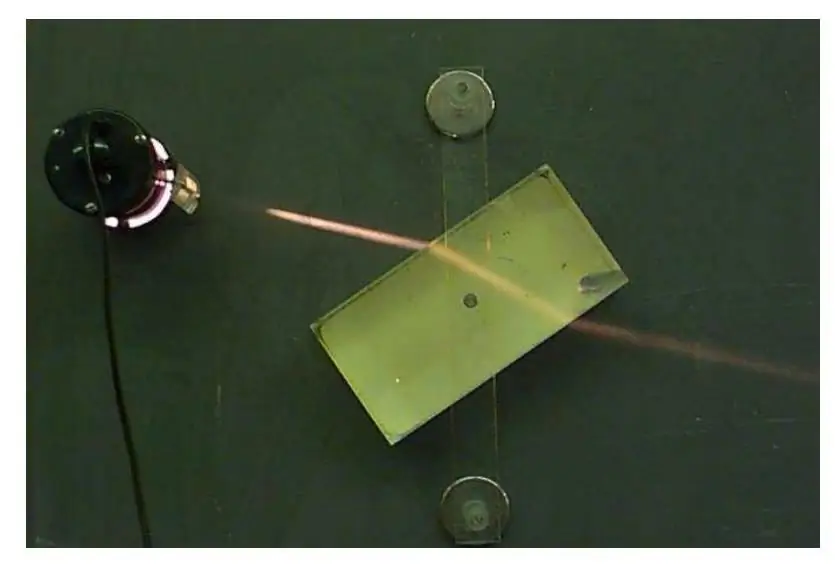
Glass is a much denser medium than air. Its absolute refractive index ranges from 1.5 to 1.66, depending on the type of glass. If we take the average value of 1.55, then the refraction of the beam at the air-glass interface can be calculated using the formula:

sin(θ₁)/sin(θ₂)=n₂/ n₁=n₂₁=1, 55.

The value n₂₁ is called the relative refractive index of air - glass. If the beam goes out of the glass into the air, then the following formula should be used:

sin(θ₁)/sin(θ₂)=n₂/ n₁=n₂₁=1/1, 55=0, 645.

If the angle of the refracted beam in the latter case will be equal to 90^o, then the angle of incidence corresponding to it is called critical. For the border glass - air it is:

θ₁=arcsin(0, 645)=40, 17^o.

If the beam will fall on the glass-air boundary with greater angles than 40, 17^o, then it will be reflected completely back into the glass. This phenomenon is called "total internal reflection".

The critical angle exists only when the beam moves from a dense medium (from glass to air, but not vice versa).