I think we should start with the history of such a glorious mathematical tool as differential equations. Like all differential and integral calculus, these equations were invented by Newton at the end of the 17th century. He considered this very discovery of his so important that he even encrypted the message, which today can be translated something like this: "All laws of nature are described by differential equations." This may seem like an exaggeration, but it's true. Any law of physics, chemistry, biology can be described by these equations.

Mathematicians Euler and Lagrange made a huge contribution to the development and creation of the theory of differential equations. Already in the 18th century, they discovered and developed what they are now studying in the senior courses of universities.
A new milestone in the study of differential equations began thanks to Henri Poincare. He created a "qualitative theory of differential equations", which, in combination with the theory of functions of a complex variable, made a significant contribution to the foundation of topology - the science of space and itsproperties.

What are differential equations?
Many people are afraid of one phrase "differential equation". However, in this article we will detail the whole essence of this very useful mathematical apparatus, which is actually not as complicated as it seems from the name. In order to start talking about first-order differential equations, you should first get acquainted with the basic concepts that are inherently related to this definition. And we'll start with the differential.
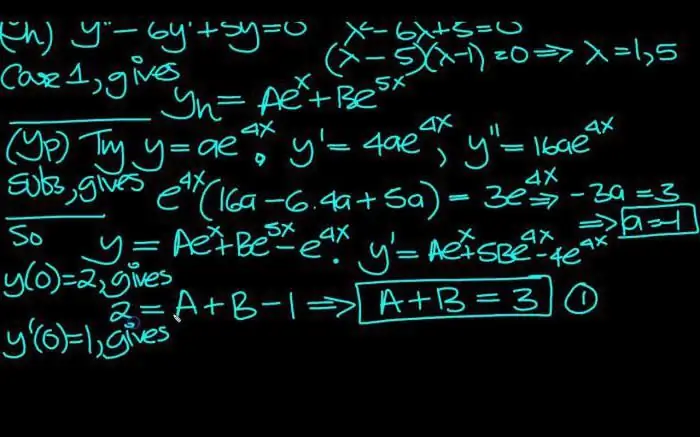
Differential
Many know this concept from school. However, let's take a closer look at it. Imagine a graph of a function. We can increase it to such an extent that any of its segments will take the form of a straight line. On it we take two points that are infinitely close to each other. The difference between their coordinates (x or y) will be an infinitesimal value. It is called a differential and is denoted by the signs dy (differential from y) and dx (differential from x). It is very important to understand that the differential is not a finite value, and this is its meaning and main function.
And now we need to consider the next element, which will be useful to us in explaining the concept of a differential equation. This is the derivative.
Derivative
We all probably heard in school and this concept. The derivative is said to be the rate of growth or decrease of a function. However, from this definitionmuch becomes unclear. Let's try to explain the derivative in terms of differentials. Let's go back to an infinitesimal segment of a function with two points that are at a minimum distance from each other. But even for this distance, the function manages to change by some amount. And to describe this change, they came up with a derivative, which can otherwise be written as a ratio of differentials: f(x)'=df/dx.
Now it is worth considering the basic properties of the derivative. There are only three of them:
- The derivative of the sum or difference can be represented as the sum or difference of derivatives: (a+b)'=a'+b' and (a-b)'=a'-b'.
- The second property is related to multiplication. The derivative of a product is the sum of the products of one function and the derivative of another: (ab)'=a'b+ab'.
- The derivative of the difference can be written as the following equality: (a/b)'=(a'b-ab')/b2.
All these properties will be useful for finding solutions to first-order differential equations.
There are also partial derivatives. Let's say we have a function z that depends on variables x and y. To calculate the partial derivative of this function, say, with respect to x, we need to take the variable y as a constant and simply differentiate.
Integral
Another important concept is the integral. In fact, this is the direct opposite of the derivative. There are several types of integrals, but to solve the simplest differential equations, we need the most trivial indefinite integrals.
So what is an integral? Let's say we have some dependency ffrom x. We take the integral from it and get the function F (x) (often called the antiderivative), the derivative of which is equal to the original function. Thus F(x)'=f(x). It also follows from this that the integral of the derivative is equal to the original function.
When solving differential equations, it is very important to understand the meaning and function of the integral, since you will have to take them very often to find a solution.
Equations are different depending on their nature. In the next section, we will consider the types of first-order differential equations, and then learn how to solve them.
Classes of differential equations
"Diffury" are divided according to the order of the derivatives involved in them. Thus, there is the first, second, third and more order. They can also be divided into several classes: ordinary and partial derivatives.
In this article we will consider ordinary differential equations of the first order. We will also discuss examples and ways to solve them in the following sections. We will consider only ODEs, because these are the most common types of equations. Ordinary are divided into subspecies: with separable variables, homogeneous and heterogeneous. Next, you will learn how they differ from each other, and learn how to solve them.
In addition, these equations can be combined, so that after we get a system of differential equations of the first order. We will also consider such systems and learn how to solve them.
Why are we considering only the first order? Because you need to start with a simple one, and describe everything related to differentialequations, in one article is simply impossible.

Separable variable equations
These are perhaps the simplest first-order differential equations. These include examples that can be written like this: y'=f(x)f(y). To solve this equation, we need a formula for representing the derivative as a ratio of differentials: y'=dy/dx. Using it, we get the following equation: dy/dx=f(x)f(y). Now we can turn to the method for solving standard examples: we will divide the variables into parts, i.e. we will transfer everything with the y variable to the part where dy is located, and we will do the same with the x variable. We get an equation of the form: dy/f(y)=f(x)dx, which is solved by taking the integrals of both parts. Do not forget about the constant that must be set after taking the integral.
The solution to any "diffurance" is a function of the dependence of x on y (in our case) or, if there is a numerical condition, then the answer is in the form of a number. Let's analyze the whole course of the solution using a specific example:
y'=2ysin(x)
Move variables in different directions:
dy/y=2sin(x)dx
Now we take integrals. All of them can be found in a special table of integrals. And we get:
ln(y)=-2cos(x) + C
If required, we can express "y" as a function of "x". Now we can say that our differential equation is solved if no condition is given. A condition can be given, for example, y(n/2)=e. Then we simply substitute the value of these variables into the solution andfind the value of the constant. In our example, it is equal to 1.
First-order homogeneous differential equations
Now on to the more difficult part. Homogeneous differential equations of the first order can be written in general form as follows: y'=z(x, y). It should be noted that the right function of two variables is homogeneous, and it cannot be divided into two dependencies: z on x and z on y. Checking whether the equation is homogeneous or not is quite simple: we make the substitution x=kx and y=ky. Now we cancel all k. If all these letters are reduced, then the equation is homogeneous and you can safely proceed to solve it. Looking ahead, let's say: the principle of solving these examples is also very simple.
We need to make a substitution: y=t(x)x, where t is some function that also depends on x. Then we can express the derivative: y'=t'(x)x+t. Substituting all this into our original equation and simplifying it, we get an example with separable variables t and x. We solve it and get the dependence t(x). When we got it, we simply substitute y=t(x)x into our previous replacement. Then we get the dependence of y on x.
To make it clearer, let's look at an example: xy'=y-xey/x.
When checking with replacement, everything is reduced. So the equation is really homogeneous. Now we make another substitution that we talked about: y=t(x)x and y'=t'(x)x+t(x). After simplification, we get the following equation: t'(x)x=-et. We solve the resulting example with separated variables and get: e-t=ln(Cx). We only need to replace t with y/x (after all, if y=tx, then t=y/x), and we getanswer: e-y/x=ln(xС).

First Order Linear Differential Equations
It's time for another big topic. We will analyze inhomogeneous differential equations of the first order. How are they different from the previous two? Let's figure it out. Linear differential equations of the first order in general form can be written as follows: y' + g(x)y=z(x). It is worth clarifying that z(x) and g(x) can be constants.
And now an example: y' - yx=x2.
There are two ways to solve it, and we will deal with both in order. The first one is the method of variation of arbitrary constants.
In order to solve the equation in this way, you must first equate the right side to zero and solve the resulting equation, which after moving the parts will take the form:
y'=yx;
dy/dx=yx;
dy/y=xdx;
ln|y|=x2/2 + C;
y=ex2/2yC=C1e x2/2.
Now we need to replace the constant C1 with the function v(x) that we have to find.
y=vex2/2.
Let's change the derivative:
y'=v'ex2/2-xvex2/2.
And substitute these expressions into the original equation:
v'ex2/2 - xvex2/2 + xvex2 /2 =x2.
You can see that two terms cancel on the left side. If in some example this did not happen, then you did something wrong. Continue:
v'ex2/2 =x2.
Now we solve the usual equation in which we need to separate the variables:
dv/dx=x2/ex2/2;
dv=x2e-x2/2dx.
To extract the integral, we have to apply integration by parts here. However, this is not the topic of our article. If you are interested, you can learn how to perform such actions yourself. It is not difficult, and with sufficient skill and attention does not take much time.
Let's turn to the second method of solving inhomogeneous equations: the Bernoulli method. Which approach is faster and easier is up to you.
So, when solving the equation by this method, we need to make a replacement: y=kn. Here k and n are some x-dependent functions. Then the derivative will look like this: y'=k'n+kn'. Substitute both substitutions into the equation:
k'n+kn'+xkn=x2.
Group:
k'n+k(n'+xn)=x2.
Now we need to equate to zero what is in brackets. Now, if you combine the two resulting equations, you get a system of first-order differential equations that you need to solve:
n'+xn=0;
k'n=x2.
The first equality is solved like a normal equation. To do this, you need to separate the variables:
dn/dx=xv;
dn/n=xdx.
Take the integral and get: ln(n)=x2/2. Then, if we express n:
n=ex2/2.
Now we substitute the resulting equality into the second equation of the system:
k'ex2/2=x2.
And transforming, we get the same equality as in the first method:
dk=x2/ex2/2.
We won't go into further steps either. It is worth saying that at first the solution of differential equations of the first order causes significant difficulties. However, as you dive deeper into the topic, it starts to get better and better.
Where are differential equations used?
Differential equations are very actively used in physics, since almost all basic laws are written in differential form, and the formulas that we see are the solution of these equations. In chemistry, they are used for the same reason: basic laws are derived from them. In biology, differential equations are used to model the behavior of systems, such as predator-prey. They can also be used to create reproduction models of, say, a colony of microorganisms.
How will differential equations help in life?
The answer to this question is simple: no way. If you are not a scientist or engineer, then they are unlikely to be useful to you. However, for general development, it does not hurt to know what a differential equation is and how it is solved. And then the question of a son or daughter "what is a differential equation?" won't confuse you. Well, if you are a scientist or an engineer, then you yourself understand the importance of this topic in any science. But the most important thing is that now the question "how to solve a first-order differential equation?" you can always answer. Agree, it's always nicewhen you understand what people are even afraid to understand.
Main learning problems
The main problem in understanding this topic is the poor skill of integrating and differentiating functions. If you are bad at taking derivatives and integrals, then you should probably learn more, master different methods of integration and differentiation, and only then start studying the material that was described in the article.
Some people are surprised when they find out that dx can be transferred, because earlier (in school) it was stated that the fraction dy/dx is indivisible. Here you need to read the literature on the derivative and understand that it is the ratio of infinitesimal quantities that can be manipulated when solving equations.
Many do not immediately realize that the solution of first-order differential equations is often a function or an integral that cannot be taken, and this delusion gives them a lot of trouble.
What else can be studied for a better understanding?
It is best to start further immersion in the world of differential calculus with specialized textbooks, for example, in calculus for students of non-mathematical speci alties. Then you can move on to more specialized literature.
It should be said that, in addition to differential equations, there are also integral equations, so you will always have something to strive for and something to study.

Conclusion
We hope that after readingThis article gave you an idea of what differential equations are and how to solve them correctly.
In any case, mathematics will somehow be useful to us in life. It develops logic and attention, without which every person is like without hands.






