It is impossible to claim that you know mathematics if you do not know how to build graphs, draw inequalities on a coordinate line, work with coordinate axes. The visual component in science is vital, because without visual examples in formulas and calculations, sometimes you can get very confused. In this article, we will see how to work with coordinate axes and learn how to build simple function graphs.
Application
The coordinate line is the basis of the simplest types of graphs that a student encounters on his educational path. It is used in almost every mathematical topic: when calculating speed and time, projecting the size of objects and calculating their area, in trigonometry when working with sines and cosines.
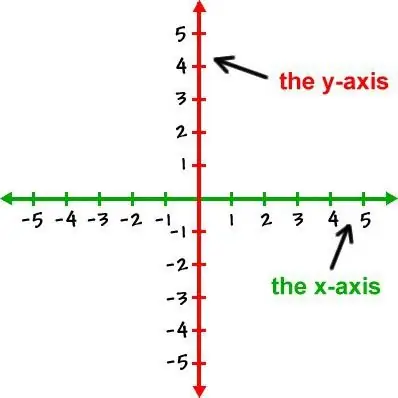
The main value of such a direct line is visibility. Since mathematics is a science that requires a high level of abstract thinking, graphs help in representing an object in the real world. How does he behave? At what point in space will thea few seconds, minutes, hours? What can be said about it in comparison with other objects? What is its speed at a randomly selected time? How to characterize his movement?
And we are talking about speed for a reason - it is often displayed by function graphs. And they can also display changes in temperature or pressure inside the object, its size, orientation relative to the horizon. Thus, building a coordinate line is often required in physics as well.
One-dimensional graph
There is a concept of multidimensionality. In one-dimensional space, just one number is enough to determine the location of a point. This is exactly the case with the use of the coordinate line. If the space is two-dimensional, then two numbers are required. Charts of this type are used much more often, and we will definitely consider them a little later in the article.
What can be seen with the help of points on the axis, if there is only one axis? You can see the size of the object, its position in space relative to some "zero", i.e. the point chosen as the reference point.
Change of parameters over time will not be visible, as all readings will be displayed for one particular moment. However, you have to start somewhere! So let's get started.
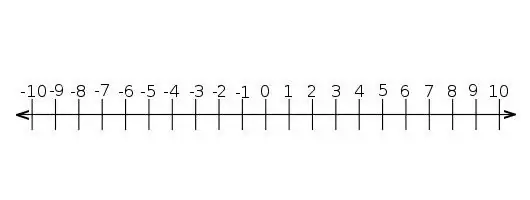
How to build a coordinate axis
First, you need to draw a horizontal line - this will be our axis. On the right side, “sharpen” it so that it looks like an arrow. Thus, we will indicate the direction in which the numbers will beincrease. In the downward direction, the arrow is usually not placed. Traditionally, the axis points to the right, so we'll just follow this rule.
Let's set a zero mark, which will display the origin of coordinates. This is the very place from which the countdown is taken, whether it be size, weight, speed, or anything else. In addition to zero, we must necessarily designate the so-called division price, i.e., introduce a unit standard, in accordance with which we will plot certain quantities on the axis. This must be done in order to be able to find the length of the segment on the coordinate line.
After an equal distance from each other, put dots or “notches” on the line, and under them write 1, 2, 3, respectively, and so on. And now, everything is ready. But with the resulting schedule, you still need to learn how to work.
Types of points on the coordinate line
From the first glance at the drawings proposed in the textbooks, it becomes clear: the points on the axis can be filled or not filled. Do you think it's a coincidence? Not at all! A "solid" dot is used for a non-strict inequality - one that reads as "greater than or equal to". If we need to strictly limit the interval (for example, "x" can take values from zero to one, but does not include it), we will use a "hollow" point, that is, in fact, a small circle on the axis. It should be noted that students do not really like strict inequalities, because they are more difficult to work with.
Depending on which points youuse on the chart, the built intervals will also be called. If the inequality on both sides is not strict, then we get a segment. If on the one hand it turns out to be “open”, then it will be called a half-interval. Finally, if a part of a line is bounded on both sides by hollow points, it will be called an interval.
Plane
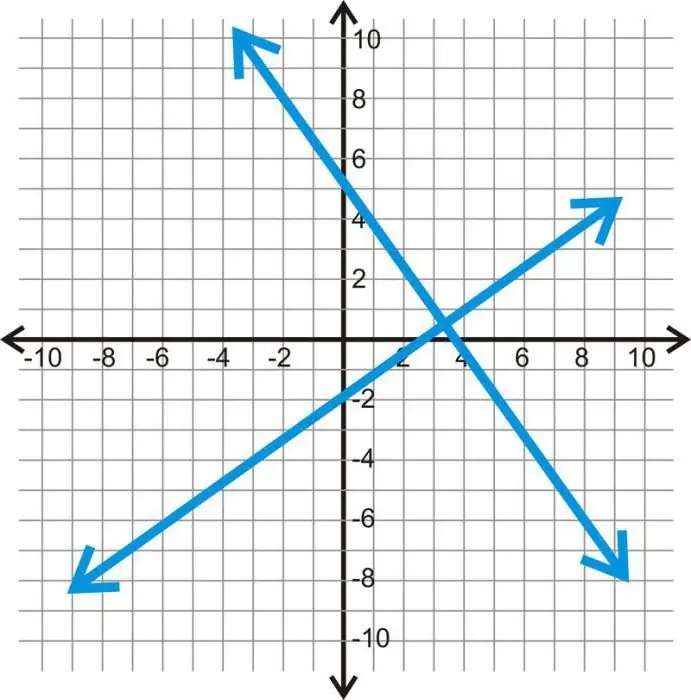
When constructing two straight lines on the coordinate plane, we can already consider the graphs of functions. Let's say the horizontal line is the time axis, and the vertical line is the distance. And now we are able to determine what distance the object will overcome in a minute or an hour of travel. Thus, working with a plane makes it possible to monitor the change in the state of an object. This is much more interesting than exploring a static state.
The simplest graph on such a plane is a straight line, it reflects the function Y(X)=aX + b. Does the line bend? This means that the object changes its characteristics during the study.
Imagine you are standing on the roof of a building holding a stone in your outstretched hand. When you release it, it will fly down, starting its movement from zero speed. But in a second he will overcome 36 kilometers per hour. The stone will continue to accelerate further, and in order to draw its movement on the chart, you will need to measure its speed at several points in time by setting points on the axis in the appropriate places.
Marks on the horizontal coordinate line by default are named X1, X2, X3, and on the vertical - Y1, Y2, Y3, respectively. Projectingthem to the plane and finding intersections, we find fragments of the resulting pattern. Connecting them with one line, we get a graph of the function. In the case of a falling stone, the quadratic function will look like: Y(X)=aXX + bX + c.
Scale
Of course, it is not necessary to put integer values next to divisions by a straight line. If you are considering the movement of a snail that crawls at a speed of 0.03 meters per minute, set as values on the coordinate fraction. In this case, set the scale interval to 0.01 meters.
It is especially convenient to carry out such drawings in a notebook in a cage - here you can immediately see if there is enough space on the sheet for your chart, if you go beyond the margins. It is not difficult to calculate your strength, because the width of the cell in such a notebook is 0.5 centimeters. It took - reduced the picture. Changing the chart scale will not cause it to lose or change its properties.
Point and segment coordinates
When a math problem is given in a lesson, it can contain the parameters of various geometric shapes, both in the form of side lengths, perimeter, area, and in the form of coordinates. In this case, you may need to both build a shape and get some data associated with it. The question arises: how to find the required information on the coordinate line? And how to build a shape?

For example, we are talking about a point. Then a capital letter will appear in the condition of the problem, and several numbers will appear in brackets, most often two (this means we will count in two-dimensional space). If there are three numbers in brackets, separated by a semicolon or a comma, then this is a three-dimensional space. Each of the values is a coordinate on the corresponding axis: first along the horizontal (X), then along the vertical (Y).
Remember how to draw a segment? You passed it on geometry. If there are two points, then a line can be drawn between them. Their coordinates are indicated in brackets if a segment appears in the problem. For example: A(15, 13) - B(1, 4). To build such a line, you need to find and mark points on the coordinate plane, and then connect them. That's it!
And any polygons, as you know, can be drawn using segments. Problem solved.
Calculations
Let's say there is some object whose position along the X axis is characterized by two numbers: it starts at the point with coordinate (-3) and ends at (+2). If we want to know the length of this object, then we must subtract the smaller number from the larger number. Note that a negative number absorbs the sign of the subtraction, because "a minus times a minus equals a plus." So we add (2+3) and get 5. This is the required result.
Another example: we are given the end point and length of the object, but not the start point (and we need to find it). Let the position of the known point be (6), and the size of the object under study be (4). By subtracting the length from the final coordinate, we get the answer. Total: (6 - 4)=2.
Negative numbers
It is often required in practice to work with negative values. In this case we willmove to the left along the coordinate axis. For example, an object 3 centimeters high floats in water. One-third of it is immersed in liquid, two-thirds is in air. Then, choosing the water surface as the axis, we get two numbers using the simplest arithmetic calculations: the top point of the object has the coordinate (+2), and the bottom one - (-1) centimeter.
It is easy to see that in the case of a plane, we have four quarters of the coordinate line. Each of them has its own number. In the first (upper right) part there will be points having two positive coordinates, in the second - on the top left - the values \u200b\u200bof the X axis will be negative, and along the Y axis - positive. The third and fourth are counted further counterclockwise.
Important property
You know that a line can be represented as an infinite number of points. We can view as carefully as we like any number of values in each direction of the axis, but we will not meet repeating ones. It seems naive and understandable, but that statement stems from an important fact: each number corresponds to one and only one point on the coordinate line.
Conclusion
Remember that any axes, figures and, if possible, graphics must be built on a ruler. Units of measurement were not invented by man by chance - if you make an error when drawing, you run the risk of seeing a different image than it should have been.
Be careful and accurate in plotting and calculations. Like any science studied in school, mathematics loves accuracy. Put in a little effort and goodevaluations will not be long in coming.






