One of the most important sciences, the application of which can be seen in disciplines such as chemistry, physics and even biology, is mathematics. The study of this science allows you to develop some mental qualities, improve abstract thinking and the ability to concentrate. One of the topics that deserve special attention in the course "Mathematics" is the addition and subtraction of fractions. Many students find it difficult to study. Perhaps our article will help to better understand this topic.
How to subtract fractions with the same denominators
Fractions are the same numbers with which you can perform various actions. Their difference from integers lies in the presence of a denominator. That is why when performing actions with fractions, you need to study some of their features and rules. The simplest case is the subtraction of ordinary fractions, the denominators of which are represented as the same number. It will not be difficult to perform this action if you know a simple rule:
In order to subtract the second from one fraction, it is necessary to subtract the numerator of the subtracted fraction from the numerator of the reduced fraction. This iswe write the number into the numerator of the difference, and leave the denominator the same: k/m - b/m=(k-b)/m
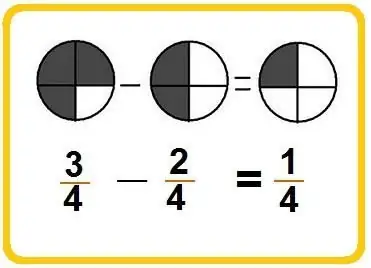
Examples of subtracting fractions whose denominators are the same
Let's see how it looks like on an example:
7/19 - 3/19=(7 - 3)/19=4/19.
From the numerator of the reduced fraction "7" subtract the numerator of the subtracted fraction "3", we get "4". We write this number in the numerator of the answer, and put in the denominator the same number that was in the denominators of the first and second fractions - “19”.
The picture below shows a few more similar examples.
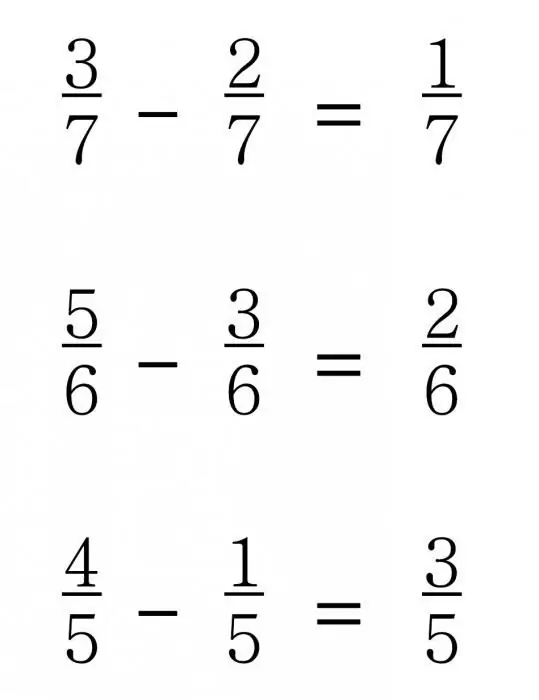
Let's consider a more complicated example where fractions with the same denominators are subtracted:
29/47 - 3/47 - 8/47 - 2/47 - 7/47=(29 - 3 - 8 - 2 - 7)/47=9/47.
From the numerator of the reduced fraction "29" by subtracting in turn the numerators of all subsequent fractions - "3", "8", "2", "7". As a result, we get the result "9", which we write in the numerator of the answer, and in the denominator we write the number that is in the denominators of all these fractions - "47".
Adding fractions with the same denominator
Addition and subtraction of ordinary fractions is carried out according to the same principle.
To add fractions with the same denominators, you need to add the numerators. The resulting number is the numerator of the sum, and the denominator remains the same: k/m + b/m=(k + b)/m
Let's see how it looks like on an example:
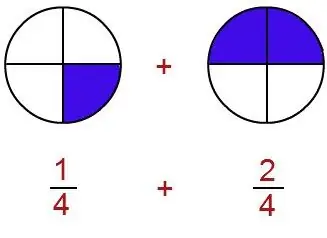
1/4 + 2/4=3/4.
Kthe numerator of the first term of the fraction - "1" - add the numerator of the second term of the fraction - "2". The result - "3" - is written in the numerator of the amount, and the denominator is the same as that present in the fractions - "4".
Fractions with different denominators and their subtraction
The action with fractions that have the same denominator, we have already considered. As you can see, knowing simple rules, solving such examples is quite easy. But what if you need to perform an action with fractions that have different denominators? Many high school students are confused by such examples. But even here, if you know the principle of the solution, the examples will no longer be difficult for you. There is also a rule here, without which the solution of such fractions is simply impossible.
-
To subtract fractions with different denominators, you need to reduce them to the same smallest denominator.

subtraction of fractions with different denominators
We'll talk more about how to do this.
Property of a fraction
In order to reduce several fractions to the same denominator, you need to use the main property of the fraction in the solution: after dividing or multiplying the numerator and denominator by the same number, you get a fraction equal to the given one.
So, for example, the fraction 2/3 can have such denominators as "6", "9", "12", etc., that is, it can look like any number that is a multiple of "3". After we multiply the numerator and denominator by"2", you get the fraction 4/6. After we multiply the numerator and denominator of the original fraction by "3", we get 6/9, and if we perform a similar action with the number "4", we get 8/12. In one equation, this can be written as follows:
2/3=4/6=6/9=8/12…
How to bring multiple fractions to the same denominator
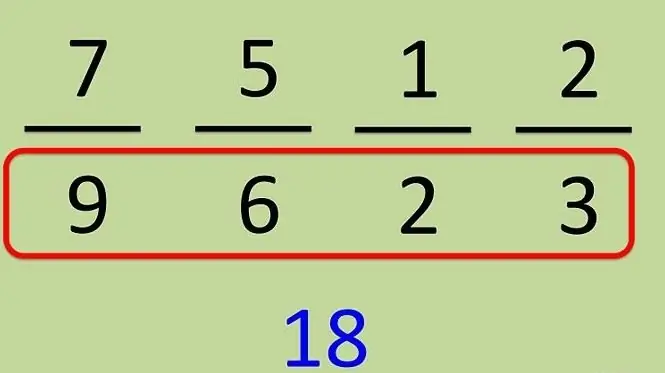
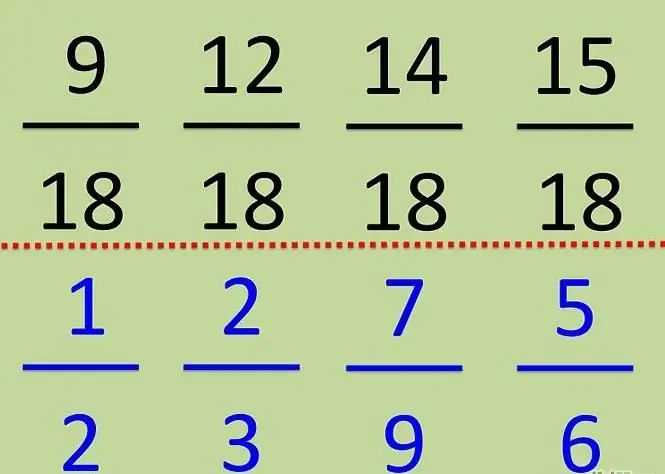
Let's consider how to reduce several fractions to the same denominator. For example, take the fractions shown in the picture below. First you need to determine what number can become the denominator for all of them. To make it easier, let's factorize the available denominators.
The denominator of the fraction 1/2 and the fraction 2/3 cannot be factored. The denominator of 7/9 has two factors 7/9=7/(3 x 3), the denominator of the fraction 5/6=5/(2 x 3). Now you need to determine which factors will be the smallest for all these four fractions. Since the first fraction has the number “2” in the denominator, it means that it must be present in all denominators, in the fraction 7/9 there are two triples, which means that they must also be present in the denominator. Given the above, we determine that the denominator consists of three factors: 3, 2, 3 and is equal to 3 x 2 x 3=18.
Consider the first fraction - 1/2. Its denominator contains "2", but there is not a single "3", but there should be two. To do this, we multiply the denominator by two triples, but, according to the property of a fraction, we must multiply the numerator by two triples:
1/2=(1 x 3 x 3) / (2 x 3 x 3)=9 /18.
Similarly, we perform actions with the remainingfractions.
-
2/3 - the denominator is missing one three and one two:
2/3=(2 x 3 x 2)/(3 x 3 x 2)=12/18.
-
7/9 or 7/(3 x 3) - the denominator is missing a denominator:
7/9=(7 x 2)/(9 x 2)=14/18.
-
5/6 or 5/(2 x 3) - the denominator is missing a triple:
5/6=(5 x 3)/(6 x 3)=15/18.
All together it looks like this:
How to subtract and add fractions with different denominators
As mentioned above, in order to add or subtract fractions with different denominators, they must be brought to the same denominator, and then use the rules for subtracting fractions with the same denominator, which have already been described.
Let's take this as an example: 4/18 - 3/15.
Find multiples of 18 and 15:
- The number 18 is 3 x 2 x 3.
- The number 15 consists of 5 x 3.
- The common multiple will consist of the following factors 5 x 3 x 3 x 2=90.
After the denominator is found, it is necessary to calculate the multiplier that will be different for each fraction, that is, the number by which it will be necessary to multiply not only the denominator, but also the numerator. To do this, we divide the number that we found (common multiple) by the denominator of the fraction for which additional factors need to be determined.
- 90 divided by 15. The resulting number "6" will be a multiplier for 3/15.
- 90 divided by 18. The resulting number "5" will be a multiplier for 4/18.
The next step in our decision isbringing each fraction to the denominator "90".
How it's done, we've already said. Consider how this is written in the example:
(4 x 5)/(18 x 5) - (3 x 6)/(15 x 6)=20/90 - 18/90=2/90=1/45.
If fractions with small numbers, then you can determine the common denominator, as in the example shown in the picture below.
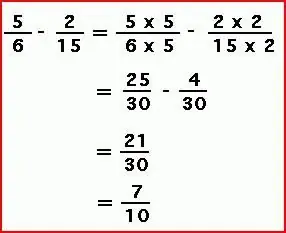
Similarly, addition of fractions with different denominators is performed.
Subtraction and addition of fractions with integer parts
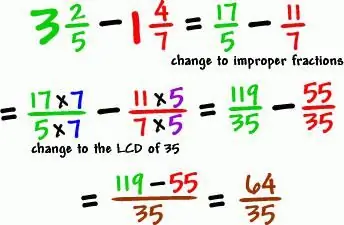
Subtraction of fractions and their addition, we have already analyzed in detail. But how to subtract if the fraction has an integer part? Again, let's use a few rules:
- Translate all fractions with an integer part into improper ones. In simple words, remove the whole part. To do this, the number of the integer part is multiplied by the denominator of the fraction, the resulting product is added to the numerator. The number that will be obtained after these actions is the numerator of an improper fraction. The denominator remains the same.
- If fractions have different denominators, they should be reduced to the same.
- Add or subtract with the same denominators.
- When receiving an improper fraction, select the integer part.
There is another way by which you can add and subtract fractions with integer parts. For this, actions are performed separately with integer parts, and separately with fractions, and the results are recorded together.
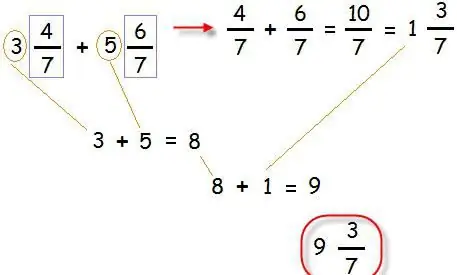
The above example consists of fractions that have the same denominator. In the case when the denominators are different, they must be reduced to the same, and then follow the steps as shown in the example.
Subtract fractions from integers
Another type of operations with fractions is the case when a fraction must be subtracted from a natural number. At first glance, such an example seems difficult to solve. However, everything is quite simple here. To solve it, it is necessary to convert an integer into a fraction, and with such a denominator, which is in the fraction to be subtracted. Next, we perform a subtraction similar to subtraction with the same denominators. In an example, it looks like this:
7 - 4/9=(7 x 9)/9 - 4/9=53/9 - 4/9=49/9.
The subtraction of fractions presented in this article (Grade 6) is the basis for solving more complex examples that are considered in subsequent classes. Knowledge of this topic is used subsequently to solve functions, derivatives, and so on. Therefore, it is very important to understand and understand the operations with fractions discussed above.






