Quadagons, as a special case of polygons, are a very important topic studied in the school geometry course. The modern program implies familiarization with this material in the eighth grade. In the framework of schooling, only convex quadrilaterals are considered. The rest are studied at the level of higher educational institutions.
The study of quadrilaterals is not the same in different programs for studying geometry. The order in which the concept is introduced depends on the sequence in which material about polygons is presented.
The order of studying quadrilaterals
In one case, a quadrilateral is considered as a special case of a polygon, in another it is defined as a set of segments and points located at their intersection, four in number. In this case, the conditions of non-belonging of any of these three points to one straight line, and the absence of intersections, except at the vertices, must be satisfied.
Most schoolsQuadrangles are studied in the eighth grade. Having first studied the parallelism of lines, then the theorem on the sum of angles of a polygon, they pass to a parallelogram. Having considered its features and proved the theorems related to them, they move on to the rest of the special cases, getting answers to the questions: what quadrilateral is called a square, a rhombus, a rectangle and various types of trapezoids.
Another approach is to study quadrilaterals when considering the topic of similar shapes. Here, quadrilaterals are also studied sequentially, starting with a parallelogram. It is determined which quadrilateral is called a rectangle, a trapezoid. And of course, it is considered in detail what other quadrangles can be.
Classification of figures with four corners
Which quadrilateral is called a square? You can find out by examining all the figures related to this one in order. The first object that comes to our attention is called a parallelogram. It is formed by four straight lines, pairwise parallel and intersecting. Separately, the cases are defined when this occurs at angles of ninety degrees and those in which all the segments formed by such intersections have the same length. Finally, let's find out which quadrilateral is called a trapezoid.
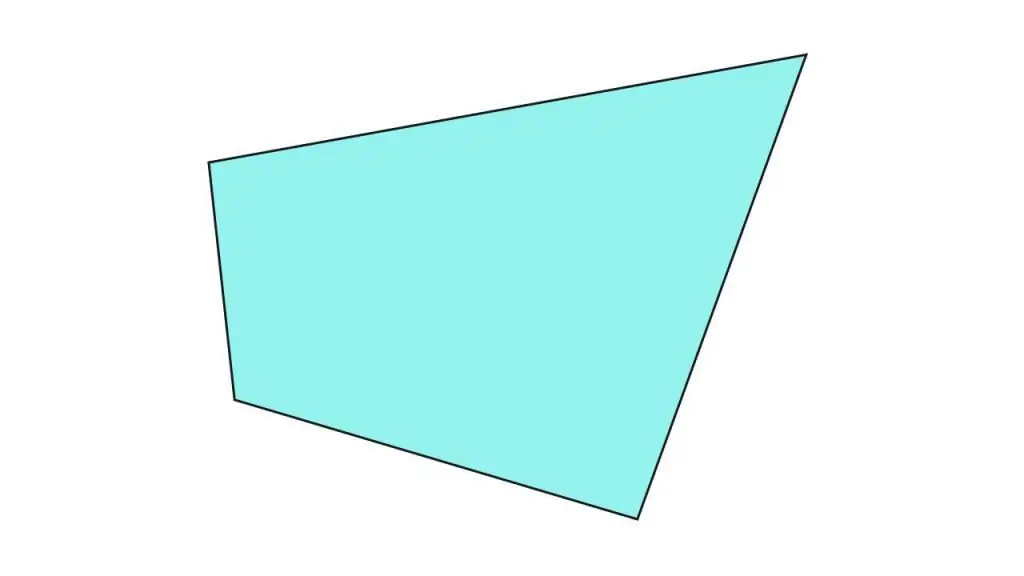
Quadangles called convex
Let's dwell on the concepts of convex and non-convex quadrilaterals. This distinction is of great importance, since only the first of them are studied in the school curriculum.
What a quadrilateralcalled convex? In order to understand this sequentially, we draw straight lines through all sides of the figure. If in all cases the entire quadrilateral lies in one of the two half-planes formed by this line, it is convex. Otherwise, respectively, non-convex.
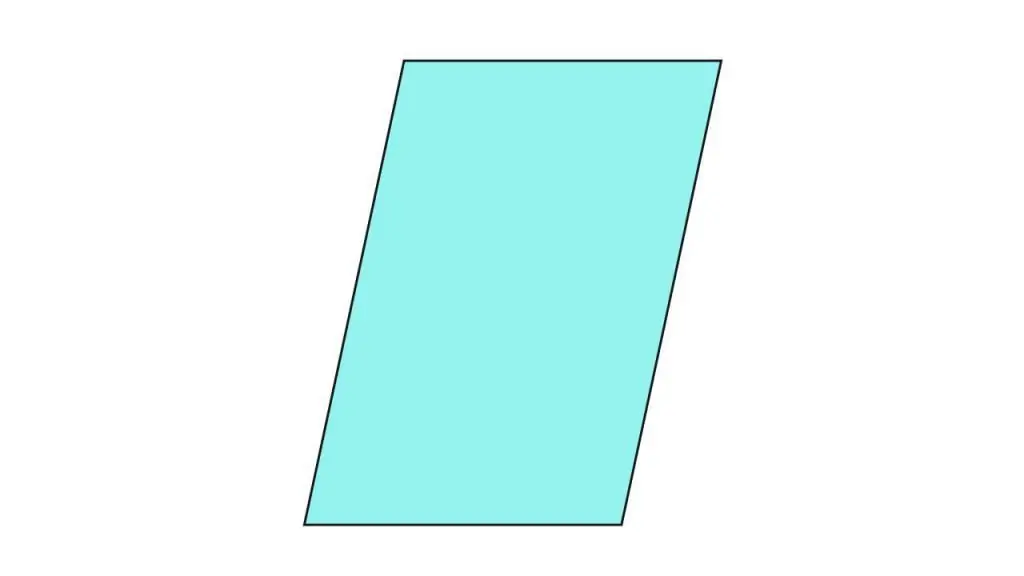
Regular parallelogram
Now consider the main types of convex quadrilaterals. Let's start with a parallelogram. Above we gave the definition of this figure. In addition to the definition, it is worth noting several properties of this convex polygon.
The sides of a parallelogram opposite each other are equal. The opposite angles are also equal to each other.
The intersection of segments called diagonals forms an angle of ninety degrees. If you sum up the squares of their lengths, then they will be the sum of the squares of the faces of the figure. Each such segment forms two identical triangles and four equal ones.
Any two adjacent angles add up to one hundred and eighty degrees.
When stating the fact that a geometric figure has these properties, it can be argued that it is a parallelogram. Thus, we will get the signs of this quadrilateral, which determine whether the figure belongs to this particular class.
The area can be found in two ways. The first will be the search for the product of the sine of the angle and the lengths of the sides adjacent to it. The second way is to determine the result of multiplying the lengths of the height and the face opposite it.

Diamond
Which quadrilateral is called a rhombus? One in which all of the sides forming it are equal to each other. This geometric figure has all the properties and features of a parallelogram. Another property is the fact that a circle is always inscribed in this figure.
A parallelogram whose adjacent sides are equal is uniquely defined as a rhombus. The area can be calculated as the product of the square of the side and the sine of one of the angles.
Rectangle
Which quadrilateral is called a rectangle? One that has ninety-degree angles. Since it is also a parallelogram, the properties and features of this quadrilateral apply to it. You can also say the following about a rectangle:
- The diagonals of this figure have the same length.
- The area is determined by multiplying the sides by each other.
- In the case when the angle of the parallelogram is ninety degrees, it can be argued that it is a rectangle.

Square
The next question from those that we will consider in this publication is what kind of quadrilateral is called a square? This is a figure with equal sides and angles of ninety degrees. Based on the above parameters, it has all the same properties that a rectangle and a rhombus have. Accordingly, it also has their signs.
The features of a square include the unique properties of the lines connecting itopposite vertices and are called diagonals. They are the same length and intersect at right angles.
The applied value of the square is difficult to overestimate. Due to its versatility, ease of determining the area and dimensions, this figure is widely used as a reference measure. A number raised to the second power is consistently called a square by mathematicians. With the help of square units, the area is measured, integration and general approximations of dimensions on the plane are carried out. This geometric concept is widely used in architecture and landscape design.

Trapezoid
Next, consider which quadrilateral is called a trapezoid. This will be a figure that has sides parallel to each other, called bases, and non-parallel sides, defined by sides. It is formed by four faces and the same number of corners. When these non-parallel segments are equal, the trapezoid is defined as isosceles. If the figure has an angle of ninety degrees, it will be considered rectangular.
Such a quadrilateral, which is called a trapezoid, has one more special element. The line that connects the centers of the sides is called the middle line. Its length can be determined by finding one half of the result of adding the lengths of the sides, defined as the base of the figure.
An isosceles trapezoid, like an isosceles triangle, has the same diagonal lengths and angles between the sides and bases.
A circle description is always possible around such a trapezoid.
A circle fits into such a figure, the sum of the lengths of the sides of which is the same as the result of adding its bases.

General conclusions on the topic
In conclusion, we can say that in the course of geometry it is quite accessible and the question of which quadrilateral is called a square is considered in detail. Despite the fact that in different textbooks we can find some differences in the sequence of presentation of the topics indicated above, all of them exhaustively cover the topic of quadrangles.






